Difference between revisions of "Conformal diagrams: stationary black holes"
(→Problem 1: Schwarzschild exterior.) |
|||
| Line 1: | Line 1: | ||
[[Category:Horizons|5]] | [[Category:Horizons|5]] | ||
| + | |||
| + | __TOC__ | ||
In the context of black hole spacetimes there are many subtly distinct notions of horizons, with the most useful being different from those used in cosmology. In particular, particle horizons do not play any role. The event horizon is defined not with respect to some selected observer, but with respect to ''all'' external observers: in an asymptotically flat spacetime'''*''' a future event horizon is the hypersurface which separates the events causally connected to future infinity and those that are not. Likewise the past event horizon delimits the events that are causally connected with past infinity or not. Another simple but powerful concept is the ''Killing horizon'': in a spacetime with a Killing vector field $\xi^\mu$ it is a (hyper-)surface, on which $\xi^\mu$ becomes lightlike. We will see explicitly for the considered examples that the Killing horizons are in fact event horizons by constructing the corresponding conformal diagrams. In general, in the frame of GR a Killing horizon in a stationary spacetime is (almost) always an event horizon and also coincides with most other notions of horizons there are. | In the context of black hole spacetimes there are many subtly distinct notions of horizons, with the most useful being different from those used in cosmology. In particular, particle horizons do not play any role. The event horizon is defined not with respect to some selected observer, but with respect to ''all'' external observers: in an asymptotically flat spacetime'''*''' a future event horizon is the hypersurface which separates the events causally connected to future infinity and those that are not. Likewise the past event horizon delimits the events that are causally connected with past infinity or not. Another simple but powerful concept is the ''Killing horizon'': in a spacetime with a Killing vector field $\xi^\mu$ it is a (hyper-)surface, on which $\xi^\mu$ becomes lightlike. We will see explicitly for the considered examples that the Killing horizons are in fact event horizons by constructing the corresponding conformal diagrams. In general, in the frame of GR a Killing horizon in a stationary spacetime is (almost) always an event horizon and also coincides with most other notions of horizons there are. | ||
| Line 59: | Line 61: | ||
</div> | </div> | ||
</div> | </div> | ||
| − | |||
| − | |||
| − | |||
| + | <div id=""></div> | ||
| + | === Problem 2: Schwarzschild interior. === | ||
| + | The region $r\in (0,r_g)$ represents the black hole's interior, between the horizon $r=r_g$ and the singularity $r=0$. Construct the conformal diagram for this region following the same scheme as before. | ||
| + | <br/> | ||
| + | '''(a)''' Which of the coordinates $(t,r)$ are timelike and which are spacelike? | ||
| + | '''(b)''' Is the singularity spacelike or timelike? | ||
| + | '''(c)''' Is the interior solution static? | ||
| + | <br/> | ||
| + | The Schwarzschild black hole's interior is an example of the ''T-region'', where $f(r)<0$, as opposed to the ''R-region'', where $f(r)>0$. | ||
<div class="NavFrame collapsed"> | <div class="NavFrame collapsed"> | ||
<div class="NavHead">solution</div> | <div class="NavHead">solution</div> | ||
<div style="width:100%;" class="NavContent"> | <div style="width:100%;" class="NavContent"> | ||
| − | <p style="text-align: left;"></p> | + | <p style="text-align: left;">The procedure is exactly the same as for the exterior region, the only thing that is different is the ranges of coordinates used and reversal of the timelike and spacelike coordinates: |
| + | <br/> | ||
| + | '''(a)''' Now $t$ is spacelike and $r$ a timelike coordinate: | ||
| + | \begin{equation} | ||
| + | ds^2 =|f(r)|^{-1}dr^2 -|f(r)|dt^2 . | ||
| + | \end{equation} | ||
| + | '''(b)''' The singularity $r=0$ is therefore a spacelike one-dimensional object: one does not "reach" it or not, instead one lives until the specified time. | ||
| + | '''(c)''' The interior solution in not static, as the metric essentially depends on the new time coordinate $r$ (or $-r$). | ||
| + | |||
| + | The null coordinates then are (note the second sign) | ||
| + | \begin{equation} | ||
| + | v=t+x,\qquad u=x-t. | ||
| + | \end{equation} | ||
| + | The horizon is at $v\to -\infty$ when $u=const$ and at $u\to -\infty$ when $v=const$. After shrinking with $\arctan$ it is pulled to $v=-\pi/2$ and $u=-\pi/2$. The third part of the boundary, corresponding to the singularity $r=0$, is $v+u=const$. | ||
| + | |||
| + | Finally, we return to timelike and spacelike coordinates by introducing (there are two ways of choosing the sign) | ||
| + | \begin{equation} | ||
| + | T=\pm (v+u),\qquad R=V-U. | ||
| + | \end{equation} | ||
| + | The resulting conformal diagram is a 45 degrees right triangle with the right angle pointing either up (then the singularity is in the past) or down (then the singularity is in future). Note that we will know which of the horizons is past and which is future only when we match the blocks with the ones that have the corresponding infinities (although one can guess already where those will be). | ||
| + | |||
| + | {|class="wikitable" style="margin: 1em auto 1em auto;" | ||
| + | |[[File:Conf-BH-SchwInt.png|center|thumb|365px|Conformal diagram for the interior region of Schwarzschild with future singularity. The dashed part of the boundary is the horizon(s); dash-dotted thin lines represent null geodesics; thin red lines are lines of constant $r$, thin blue lines are lines of constant $t$..]] | ||
| + | |} | ||
| + | </p> | ||
</div> | </div> | ||
</div> | </div> | ||
<div id=""></div> | <div id=""></div> | ||
| − | === Problem | + | === Problem 3: Geodesic incompleteness and horizon regularity. === |
| − | + | Consider radial motion of a massive particle and show that the exterior and interior parts of the Schwarzschild are not by themselves geodesically complete, i.e. particle's worldlines are terminated at the horizon at finite values of affine parameter. Show that, on the other hand, the horizon is not a singularity, by constructing the null coordinate frame, in which the metric on the horizon is explicitly regular. | |
<div class="NavFrame collapsed"> | <div class="NavFrame collapsed"> | ||
<div class="NavHead">solution</div> | <div class="NavHead">solution</div> | ||
<div style="width:100%;" class="NavContent"> | <div style="width:100%;" class="NavContent"> | ||
| − | <p style="text-align: left;"></p> | + | <p style="text-align: left;">The geodesic equation is obtained from normalization condition |
| + | \begin{equation} | ||
| + | \varepsilon^2=u^\mu u_\mu | ||
| + | =f(r)\Big(\frac{dt}{d\lambda}\Big)^{2} | ||
| + | -f^{-1}(r) | ||
| + | \Big(\frac{dr}{d\lambda}\Big)^{2}, | ||
| + | \end{equation} | ||
| + | where $\varepsilon^2=0$ or $\varepsilon^2=1$ for null and timelike geodesics respectively, and conservation equation due to the Killing vector | ||
| + | \begin{equation} | ||
| + | E=u^\mu \xi_\mu =u_0 | ||
| + | =f(r)\frac{dt}{d\lambda}, \label{BH-energy} | ||
| + | \end{equation} | ||
| + | and reads | ||
| + | \begin{equation} | ||
| + | \frac{d\lambda}{dr} | ||
| + | =\Big[E^2 -f(r)\varepsilon^2 \Big]^{-1/2}. \label{BH-lambda} | ||
| + | \end{equation} | ||
| + | The integral $\lambda(r)$ converges at $r=r_g$, so the value of the affine parameter at the horizon is finite. | ||
| + | |||
| + | In regard to regularity let us start from the exterior region. In terms of $u,v$ the metric has the overall conformal factor, which turns to zero at the horizon: | ||
| + | \begin{equation} | ||
| + | f(r)\sim (r-r_g)\sim e^{\ln (r-r_g)} | ||
| + | \sim e^{x}\sim e^{(v-u)/2}, | ||
| + | \end{equation} | ||
| + | so in order to eliminate that, we just need to use new null coordinates $(u',v')$, such that the conformal factor in | ||
| + | \begin{equation} | ||
| + | ds^{2} | ||
| + | \sim \Big(e^{-u/2}\frac{du}{du'}\Big) | ||
| + | \sim \Big(e^{+v/2}\frac{dv}{dv'}\Big) | ||
| + | du' dv' | ||
| + | \end{equation} | ||
| + | is finite and does not turn to zero. This is achieved e.g. by coordinate transformation to | ||
| + | \begin{equation} | ||
| + | u'=e^{u/2},\qquad v'=e^{-v/2}. | ||
| + | \end{equation} | ||
| + | After this one can carry out the second part of construction of the conformal diagram.</p> | ||
</div> | </div> | ||
</div> | </div> | ||
<div id=""></div> | <div id=""></div> | ||
| − | === Problem | + | === Problem 4: Piecing the puzzle. === |
| + | Geodesic incompleteness means the full conformal diagram must be assembled from the parts corresponding to external and internal solutions by gluing them together along same values of $r$ (remember that each point of the diagram corresponds to a sphere). Piece the puzzle. | ||
| + | Note that a) there are two variants of both external and internal solutions' diagrams, differing with orientation and b) the boundaries of the full diagram must go along either infinities or singularities. | ||
<div class="NavFrame collapsed"> | <div class="NavFrame collapsed"> | ||
<div class="NavHead">solution</div> | <div class="NavHead">solution</div> | ||
<div style="width:100%;" class="NavContent"> | <div style="width:100%;" class="NavContent"> | ||
| − | <p style="text-align: left;"></p> | + | <p style="text-align: left;">Starting from any one of the four pieces, there is only one way to assemble the diagram, and one has to use all the parts: |
| + | |||
| + | <gallery widths=300px heights=300px perrow=2 caption="The puzzle pieces: two for the exterior region (above) and two for the interior region (below).} | ||
| + | \end{figure"> | ||
| + | File:Conf-BH-SchwExtA.png | ||
| + | File:Conf-BH-SchwExtB.png | ||
| + | File:Conf-BH-SchwIntA.png | ||
| + | File:Conf-BH-SchwIntB.png | ||
| + | </gallery> | ||
| + | |||
| + | The horizon structure is very similar to that of full de Sitter spacetime in open slicing or ``static'' coordinates (the notions and properties of R- and T-regions apply equally well), while the structure of infinity is the same as that of Minkowski, and additionally there are singularities. | ||
| + | |||
| + | Finally, one could note that the form of the conformal block for the exterior solution was determined from the start. First, we have asymptotic flatness, therefore the Minkowski's structure of infinity. This is already half of the block's boundary. | ||
| + | |||
| + | {|class="wikitable" style="margin: 1em auto 1em auto;" | ||
| + | |[[File:Conf-BH-SchwFull.png|center|thumb|365px|{The full conformal diagram for the (maximally extended) Schwarzschild solution.} | ||
| + | \end{figure]] | ||
| + | |} | ||
| + | </p> | ||
</div> | </div> | ||
</div> | </div> | ||
| − | + | <!-- | |
<div id=""></div> | <div id=""></div> | ||
=== Problem 1 === | === Problem 1 === | ||
Revision as of 22:52, 3 February 2014
Contents
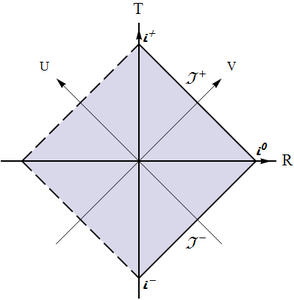
In the context of black hole spacetimes there are many subtly distinct notions of horizons, with the most useful being different from those used in cosmology. In particular, particle horizons do not play any role. The event horizon is defined not with respect to some selected observer, but with respect to all external observers: in an asymptotically flat spacetime* a future event horizon is the hypersurface which separates the events causally connected to future infinity and those that are not. Likewise the past event horizon delimits the events that are causally connected with past infinity or not. Another simple but powerful concept is the Killing horizon: in a spacetime with a Killing vector field $\xi^\mu$ it is a (hyper-)surface, on which $\xi^\mu$ becomes lightlike. We will see explicitly for the considered examples that the Killing horizons are in fact event horizons by constructing the corresponding conformal diagrams. In general, in the frame of GR a Killing horizon in a stationary spacetime is (almost) always an event horizon and also coincides with most other notions of horizons there are.
This section elaborates on the techniques of constructing conformal diagrams for stationary black hole solutions. The construction for the Schwarzschild black hole, or its variation, can be found in most textbooks on GR; for the general receipt see \cite{BrRubin}.
* Meaning the spacetime possesses the infinity with the same structure as that of Minkowski, which is important.
Schwarzschild-Kruskal black hole solution
Problem 1: Schwarzschild exterior.
The simplest black hole solution is that of Schwarzschild, given by
\begin{equation}
ds^{2}=f(r)dt^2 -\frac{dr^2}{f(r)}-r^2 d\Omega^2,\qquad f(r)=1-\frac{r_g}{r},
\label{SchwBH}
\end{equation}
where $r_g$ is the gravitational radius, and $d\Omega^2$ is the angular part of the metric, which we will not be concerned with. The surface $r=r_g$ is the horizon. Focus for now only on the external part of the solution,
\[\big\{-\infty<t<+\infty,\;\;
r_g<r<+\infty)\big\}.\]
The general procedure of building a conformal diagram for the $(t,r)$ slice, as discussed in the cosmological context earlier, works here perfectly well, but needs one additional step in the beginning:
(a) use a new radial coordinate to bring the metric to conformally flat form;
(b) pass to null coordinates;
(c) shrink the ranges of coordinate values to finite intervals with the help of $\arctan$;
(d) return to timelike and spacelike coordinates.
Identify the boundaries of Schwarzschild's exterior region on the conformal diagram and compare it with Minkowski spacetime's.
(a) In terms of the new ("tortoise") coordinate
\begin{equation}
x=\int \frac{dr}{f(r)}=r+r_g \ln |r-r_g |
\end{equation}
we get
\begin{equation}
ds^2 =f(r(x))\big[dt^2 -dx^2\big].
\end{equation}
The horizon is pushed to infinity: $x\to -\infty$.
(b) Introduce
\begin{equation}
v=t+x,\quad u=t-x
\end{equation}
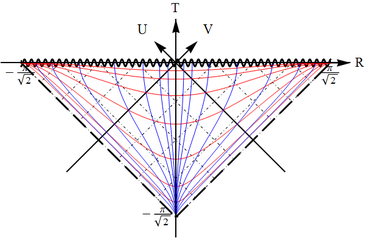
The horizon is at $v\to-\infty$ when $u=const$ (past horizon) and at $u\to -\infty$ when $v=const$ (future horizon). The asymptotically flat infinity is in the opposite direction: at $v\to+\infty$ when $u=const$ and at $u\to +\infty$ when $v=const$
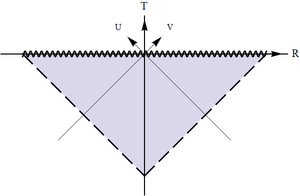
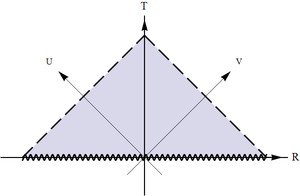
(c) Pass to $V=\arctan v$ and $U=\arctan u$; the horizon now becomes two lines at $V=-\pi/2$ and $U=-\pi/2$. The infinity is $V=+\pi/2$ and $U=+\pi/2$. The full exterior region is the square enclosed by those four lines.
(d) Transformation to $T=V+U$, $R=V-U$ rotates this square by $\pi/4$ and this is the conformal diagram block that we need. There is a past and future horizon. Note that while past should be below and future above (we expect the spacetime to be oriented, so that the direction of future is always uniquely determined), there is no rule that says that the horizons must be on the left and infinities on the right. Thus there are two mirror-reflected variants, with the horizons on the left and on the right.
Problem 2: Schwarzschild interior.
The region $r\in (0,r_g)$ represents the black hole's interior, between the horizon $r=r_g$ and the singularity $r=0$. Construct the conformal diagram for this region following the same scheme as before.
(a) Which of the coordinates $(t,r)$ are timelike and which are spacelike?
(b) Is the singularity spacelike or timelike?
(c) Is the interior solution static?
The Schwarzschild black hole's interior is an example of the T-region, where $f(r)<0$, as opposed to the R-region, where $f(r)>0$.
The procedure is exactly the same as for the exterior region, the only thing that is different is the ranges of coordinates used and reversal of the timelike and spacelike coordinates:
(a) Now $t$ is spacelike and $r$ a timelike coordinate:
\begin{equation}
ds^2 =|f(r)|^{-1}dr^2 -|f(r)|dt^2 .
\end{equation}
(b) The singularity $r=0$ is therefore a spacelike one-dimensional object: one does not "reach" it or not, instead one lives until the specified time.
(c) The interior solution in not static, as the metric essentially depends on the new time coordinate $r$ (or $-r$).
The null coordinates then are (note the second sign)
\begin{equation}
v=t+x,\qquad u=x-t.
\end{equation}
The horizon is at $v\to -\infty$ when $u=const$ and at $u\to -\infty$ when $v=const$. After shrinking with $\arctan$ it is pulled to $v=-\pi/2$ and $u=-\pi/2$. The third part of the boundary, corresponding to the singularity $r=0$, is $v+u=const$.
Finally, we return to timelike and spacelike coordinates by introducing (there are two ways of choosing the sign)
\begin{equation}
T=\pm (v+u),\qquad R=V-U.
\end{equation}
The resulting conformal diagram is a 45 degrees right triangle with the right angle pointing either up (then the singularity is in the past) or down (then the singularity is in future). Note that we will know which of the horizons is past and which is future only when we match the blocks with the ones that have the corresponding infinities (although one can guess already where those will be).
Problem 3: Geodesic incompleteness and horizon regularity.
Consider radial motion of a massive particle and show that the exterior and interior parts of the Schwarzschild are not by themselves geodesically complete, i.e. particle's worldlines are terminated at the horizon at finite values of affine parameter. Show that, on the other hand, the horizon is not a singularity, by constructing the null coordinate frame, in which the metric on the horizon is explicitly regular.
The geodesic equation is obtained from normalization condition \begin{equation} \varepsilon^2=u^\mu u_\mu =f(r)\Big(\frac{dt}{d\lambda}\Big)^{2} -f^{-1}(r) \Big(\frac{dr}{d\lambda}\Big)^{2}, \end{equation} where $\varepsilon^2=0$ or $\varepsilon^2=1$ for null and timelike geodesics respectively, and conservation equation due to the Killing vector \begin{equation} E=u^\mu \xi_\mu =u_0 =f(r)\frac{dt}{d\lambda}, \label{BH-energy} \end{equation} and reads \begin{equation} \frac{d\lambda}{dr} =\Big[E^2 -f(r)\varepsilon^2 \Big]^{-1/2}. \label{BH-lambda} \end{equation} The integral $\lambda(r)$ converges at $r=r_g$, so the value of the affine parameter at the horizon is finite. In regard to regularity let us start from the exterior region. In terms of $u,v$ the metric has the overall conformal factor, which turns to zero at the horizon: \begin{equation} f(r)\sim (r-r_g)\sim e^{\ln (r-r_g)} \sim e^{x}\sim e^{(v-u)/2}, \end{equation} so in order to eliminate that, we just need to use new null coordinates $(u',v')$, such that the conformal factor in \begin{equation} ds^{2} \sim \Big(e^{-u/2}\frac{du}{du'}\Big) \sim \Big(e^{+v/2}\frac{dv}{dv'}\Big) du' dv' \end{equation} is finite and does not turn to zero. This is achieved e.g. by coordinate transformation to \begin{equation} u'=e^{u/2},\qquad v'=e^{-v/2}. \end{equation} After this one can carry out the second part of construction of the conformal diagram.
Problem 4: Piecing the puzzle.
Geodesic incompleteness means the full conformal diagram must be assembled from the parts corresponding to external and internal solutions by gluing them together along same values of $r$ (remember that each point of the diagram corresponds to a sphere). Piece the puzzle.
Note that a) there are two variants of both external and internal solutions' diagrams, differing with orientation and b) the boundaries of the full diagram must go along either infinities or singularities.
Starting from any one of the four pieces, there is only one way to assemble the diagram, and one has to use all the parts:
- The puzzle pieces: two for the exterior region (above) and two for the interior region (below).} \end{figure
The horizon structure is very similar to that of full de Sitter spacetime in open slicing or ``static coordinates (the notions and properties of R- and T-regions apply equally well), while the structure of infinity is the same as that of Minkowski, and additionally there are singularities.
Finally, one could note that the form of the conformal block for the exterior solution was determined from the start. First, we have asymptotic flatness, therefore the Minkowski's structure of infinity. This is already half of the block's boundary.