Difference between revisions of "Solutions of Friedman equations in the Big Bang model"
(→Problem 31.) |
(→Problem 18: age of the Universe) |
||
| (51 intermediate revisions by 2 users not shown) | |||
| Line 1: | Line 1: | ||
| − | [[Category:Dynamics of the Universe in the Big Bang Model]] | + | [[Category:Dynamics of the Universe in the Big Bang Model|2]] |
| + | __TOC__ | ||
| + | == One component dominated Universe== | ||
<div id="dyn5"></div> | <div id="dyn5"></div> | ||
| − | === Problem 1 | + | <div style="border: 1px solid #AAA; padding:5px;"> |
| − | + | === Problem 1: matter or radiation in a flat Universe === | |
Derive $\rho(a)$, $\rho(t)$ and $ a(t)$ for a spatially flat$^*$ ($k=0$) Universe that consists of only | Derive $\rho(a)$, $\rho(t)$ and $ a(t)$ for a spatially flat$^*$ ($k=0$) Universe that consists of only | ||
| Line 50: | Line 52: | ||
Note that the dependence $\rho(t)$ is the same in both cases.</p> | Note that the dependence $\rho(t)$ is the same in both cases.</p> | ||
</div> | </div> | ||
| + | </div> | ||
</div> | </div> | ||
| − | |||
| − | === Problem 2 | + | <div id="dyn6"></div> |
| − | Consider two spatially flat Universes. One is filled with radiation, the other with dust. The current energy density is the same. Compare energy densities when both of them are twice as old. | + | <div style="border: 1px solid #AAA; padding:5px;"> |
| + | === Problem 2: RD and MD regimes === | ||
| + | Consider two spatially flat Universes. One is filled with radiation (thus radiation dominated, or RD), the other with dust (thus matter dominated, MD). The current energy density is the same. Compare energy densities when both of them are twice as old. | ||
<div class="NavFrame collapsed"> | <div class="NavFrame collapsed"> | ||
<div class="NavHead">solution</div> | <div class="NavHead">solution</div> | ||
| Line 65: | Line 69: | ||
is the current age of the Universe. When both Universes are twice older, density will be four times smaller in each of them.</p> | is the current age of the Universe. When both Universes are twice older, density will be four times smaller in each of them.</p> | ||
</div> | </div> | ||
| + | </div> | ||
</div> | </div> | ||
| + | |||
<div id="dyn11"></div> | <div id="dyn11"></div> | ||
| − | === Problem 3 | + | <div style="border: 1px solid #AAA; padding:5px;"> |
| + | === Problem 3: RD$\to$MD transition === | ||
Find the scale factor and density of each component as functions of time in a flat Universe which consists of dust and radiation, for the case one of the components is dominating. Present the results graphically. | Find the scale factor and density of each component as functions of time in a flat Universe which consists of dust and radiation, for the case one of the components is dominating. Present the results graphically. | ||
<div class="NavFrame collapsed"> | <div class="NavFrame collapsed"> | ||
<div class="NavHead">solution</div> | <div class="NavHead">solution</div> | ||
| − | <div style="width:100%;" class="NavContent"> | + | <div style="width:100%;" class="NavContent"><gallery widths=600px heights=500px> |
| − | + | File:3_11.jpg|Asymptotic behavior of $\rho(t)$ for the case of background interaction of matter and radiation. | |
| − | If matter and radiation do not interact, as is assumed, we can write down energy conservation laws for them separately. Then for radiation $\rho_{r}\sim a^{-4}$ and for matter $\rho_{m}\sim a^{-3}$, so energy density of radiation in an expanding Universe always decreases faster than that of non-relativistic matter. Thus the dynamics of the Universe can be divided into two stages: the first one when the dominating component is radiation, and the second one when the dominating component is non-relativistic matter. | + | </gallery> |
| − | + | <p style="text-align: left;">If matter and radiation do not interact, as is assumed, we can write down energy conservation laws for them separately. Then for radiation $\rho_{r}\sim a^{-4}$ and for matter $\rho_{m}\sim a^{-3}$, so energy density of radiation in an expanding Universe always decreases faster than that of non-relativistic matter. Thus the dynamics of the Universe can be divided into two stages: the first one when the dominating component is radiation, and the second one when the dominating component is non-relativistic matter. | |
| + | <br/> | ||
The first stage: radiation domination. The first Friedman equation is | The first stage: radiation domination. The first Friedman equation is | ||
\[\left(\frac{\dot a}{a}\right)^2 | \[\left(\frac{\dot a}{a}\right)^2 | ||
| Line 83: | Line 91: | ||
\approx\frac{8\pi G}{3}\rho_r.\] | \approx\frac{8\pi G}{3}\rho_r.\] | ||
Then $a(t)\sim t^{1/2}$ and $\rho_r \sim t^{-2}.$ | Then $a(t)\sim t^{1/2}$ and $\rho_r \sim t^{-2}.$ | ||
| − | + | <br/> | |
The dominating component determines the behavior of scale factor as function of time. Therefore we have to plug $a(t)$ for the Universe with dominating radiation into $\rho_m = \rho_m(a)$. Then $\rho_m \sim a^{-3} \sim t^{3/2}$. The relative density of matter increases with time, so the stage of radiation domination is succeeded by the stage of matter domination. | The dominating component determines the behavior of scale factor as function of time. Therefore we have to plug $a(t)$ for the Universe with dominating radiation into $\rho_m = \rho_m(a)$. Then $\rho_m \sim a^{-3} \sim t^{3/2}$. The relative density of matter increases with time, so the stage of radiation domination is succeeded by the stage of matter domination. | ||
| − | + | <br/> | |
Stage 2: matter domination. Following the same train of thought we get | Stage 2: matter domination. Following the same train of thought we get | ||
\[ a \sim t^{2/3};\quad\rho _m \sim \frac{1}{t^2}; | \[ a \sim t^{2/3};\quad\rho _m \sim \frac{1}{t^2}; | ||
| Line 91: | Line 99: | ||
\sim \frac{1}{t^{8/3}}.\]</p> | \sim \frac{1}{t^{8/3}}.\]</p> | ||
</div> | </div> | ||
| − | </div> | + | </div></div> |
| + | |||
<div id="dyn17"></div> | <div id="dyn17"></div> | ||
| − | === Problem 4 | + | <div style="border: 1px solid #AAA; padding:5px;"> |
| + | === Problem 4: Hubble parameter === | ||
Derive the time dependence of the Hubble parameter for a flat Universe in which either matter or radiation is dominating. | Derive the time dependence of the Hubble parameter for a flat Universe in which either matter or radiation is dominating. | ||
<div class="NavFrame collapsed"> | <div class="NavFrame collapsed"> | ||
<div class="NavHead">solution</div> | <div class="NavHead">solution</div> | ||
<div style="width:100%;" class="NavContent"> | <div style="width:100%;" class="NavContent"> | ||
| − | <p style="text-align: left;">a) matter domination | + | <p style="text-align: left;">'''a)''' matter domination |
$H \equiv\dot a/a = 2/(3t).$ | $H \equiv\dot a/a = 2/(3t).$ | ||
| − | + | <br/> | |
| − | b) radiation domination | + | '''b)''' radiation domination |
$H =1/(2t).$</p> | $H =1/(2t).$</p> | ||
</div> | </div> | ||
| − | </div> | + | </div></div> |
| + | |||
| + | |||
| + | == Matter and radiation == | ||
<div id="dyn12"></div> | <div id="dyn12"></div> | ||
| − | === Problem 5 | + | <div style="border: 1px solid #AAA; padding:5px;"> |
| + | === Problem 5: exact solutions for matter and radiation === | ||
Derive the exact solutions of the Friedman equations for the Universe filled with matter and radiation. Plot the graphs of scale factor and both densities as functions of time. | Derive the exact solutions of the Friedman equations for the Universe filled with matter and radiation. Plot the graphs of scale factor and both densities as functions of time. | ||
<div class="NavFrame collapsed"> | <div class="NavFrame collapsed"> | ||
<div class="NavHead">solution</div> | <div class="NavHead">solution</div> | ||
| − | <div style="width:100%;" class="NavContent | + | <div style="width:100%;" class="NavContent"><gallery widths=400px heights=300px> |
| − | + | ||
File:3_12.jpg|Scale factor $a(t)$ for a flat Universe filled with dust and radiation. | File:3_12.jpg|Scale factor $a(t)$ for a flat Universe filled with dust and radiation. | ||
File:3_12_w.jpg|Scale factor $a(t)$ for a flat Universe filled with dust and radiation. | File:3_12_w.jpg|Scale factor $a(t)$ for a flat Universe filled with dust and radiation. | ||
</gallery> | </gallery> | ||
| + | <p style="text-align: left;"> | ||
Let us present the total energy density as the sum $\rho=\rho_m+\rho _r$ and plug it into the first Friedman equation | Let us present the total energy density as the sum $\rho=\rho_m+\rho _r$ and plug it into the first Friedman equation | ||
\[\left( \frac{\dot a}{a} \right)^2 | \[\left( \frac{\dot a}{a} \right)^2 | ||
| Line 126: | Line 140: | ||
Then | Then | ||
\[\frac{\dot a}{a}= H_0\sqrt{\Omega_{m0}\left(\frac{a_0}{a} \right)^{3} +\Omega _{r0}\left(\frac{a_0}{a} \right)^{4}},\] | \[\frac{\dot a}{a}= H_0\sqrt{\Omega_{m0}\left(\frac{a_0}{a} \right)^{3} +\Omega _{r0}\left(\frac{a_0}{a} \right)^{4}},\] | ||
| − | therefore | + | therefore |
\begin{equation}\label{dyn-MRgenSol} | \begin{equation}\label{dyn-MRgenSol} | ||
t = \frac{1}{H_0}\int\limits_{0}^{a/a_0} | t = \frac{1}{H_0}\int\limits_{0}^{a/a_0} | ||
| Line 136: | Line 150: | ||
\end{equation}</p> | \end{equation}</p> | ||
</div> | </div> | ||
| − | </div> | + | </div></div> |
| + | |||
<div id="dyn20"></div> | <div id="dyn20"></div> | ||
| − | === Problem 6 | + | <div style="border: 1px solid #AAA; padding:5px;"> |
| + | === Problem 6: equipartition time === | ||
At what moment after the Big Bang did matter's density exceed that of radiation for the first time? | At what moment after the Big Bang did matter's density exceed that of radiation for the first time? | ||
<div class="NavFrame collapsed"> | <div class="NavFrame collapsed"> | ||
| Line 153: | Line 169: | ||
\frac{\Omega _{r0}^{3/2}}{\Omega _{m0}^2}.\]</p> | \frac{\Omega _{r0}^{3/2}}{\Omega _{m0}^2}.\]</p> | ||
</div> | </div> | ||
| − | </div> | + | </div></div> |
| + | |||
<div id="dyn21"></div> | <div id="dyn21"></div> | ||
| − | === Problem 7 | + | <div style="border: 1px solid #AAA; padding:5px;"> |
| + | === Problem 7: age of the Universe === | ||
Determine the age of the Universe in which either matter or radiation has always been dominating. | Determine the age of the Universe in which either matter or radiation has always been dominating. | ||
<div class="NavFrame collapsed"> | <div class="NavFrame collapsed"> | ||
| Line 167: | Line 185: | ||
Using the observed value $H_0 \approx 7.15\cdot 10^{ - 11}\mbox{yr}^{-1}$, we get $t_0 \approx 9.3 \cdot 10^{9}\mbox{yr}$ for matter and $t_0 \approx 7 \cdot 10^{9}\mbox{yr}$ for radiation.</p> | Using the observed value $H_0 \approx 7.15\cdot 10^{ - 11}\mbox{yr}^{-1}$, we get $t_0 \approx 9.3 \cdot 10^{9}\mbox{yr}$ for matter and $t_0 \approx 7 \cdot 10^{9}\mbox{yr}$ for radiation.</p> | ||
</div> | </div> | ||
| − | </div> | + | </div></div> |
| + | |||
| + | |||
| + | == Non-interacting components, effective potential == | ||
<div id="dyn14"></div> | <div id="dyn14"></div> | ||
| − | === Problem 8 | + | <div style="border: 1px solid #AAA; padding:5px;"> |
| + | === Problem 8: constant EoS parameter === | ||
Derive the dependence $a(t)$ for a spatially flat Universe that consists of matter with equation of state $p=w\rho$, assuming that the parameter $w$ does not change throughout the evolution. | Derive the dependence $a(t)$ for a spatially flat Universe that consists of matter with equation of state $p=w\rho$, assuming that the parameter $w$ does not change throughout the evolution. | ||
<div class="NavFrame collapsed"> | <div class="NavFrame collapsed"> | ||
| Line 193: | Line 215: | ||
\end{equation}</p> | \end{equation}</p> | ||
</div> | </div> | ||
| − | </div> | + | </div></div> |
| + | |||
<div id="dyn18"></div> | <div id="dyn18"></div> | ||
| − | + | <div style="border: 1px solid #AAA; padding:5px;"> | |
| − | === Problem 9 | + | === Problem 9: $H(t)$ === |
Find the Hubble parameter as function of time for the previous problem. | Find the Hubble parameter as function of time for the previous problem. | ||
<div class="NavFrame collapsed"> | <div class="NavFrame collapsed"> | ||
| Line 205: | Line 228: | ||
\[H = \frac{\dot a}{a}= \frac{2}{3\left( {1 + w} \right)t}.\]</p> | \[H = \frac{\dot a}{a}= \frac{2}{3\left( {1 + w} \right)t}.\]</p> | ||
</div> | </div> | ||
| − | </div> | + | </div></div> |
| + | |||
<div id="dyn15"></div> | <div id="dyn15"></div> | ||
| − | === Problem 10 | + | <div style="border: 1px solid #AAA; padding:5px;"> |
| + | === Problem 10: effective potential === | ||
Using the first Friedman equation, construct the effective potential $V(a)$, which governs the one-dimensional motion of a fictitious particle with coordinate $a(t)$, for the Universe filled with several non-interacting components. | Using the first Friedman equation, construct the effective potential $V(a)$, which governs the one-dimensional motion of a fictitious particle with coordinate $a(t)$, for the Universe filled with several non-interacting components. | ||
<div class="NavFrame collapsed"> | <div class="NavFrame collapsed"> | ||
| Line 251: | Line 276: | ||
\end{equation}</p> | \end{equation}</p> | ||
</div> | </div> | ||
| − | </div> | + | </div></div> |
| + | |||
<div id="dyn16"></div> | <div id="dyn16"></div> | ||
| − | + | <div style="border: 1px solid #AAA; padding:5px;"> | |
| − | === Problem 11 | + | === Problem 11: effective potential for radiation and dust === |
Construct the effective one-dimensional potential $V(a)$ (using the notation of the previous problem) for the Universe consisting of non-relativistic matter and radiation. Show that motion with $\dot{a}>0$ in such a potential can only be decelerating. | Construct the effective one-dimensional potential $V(a)$ (using the notation of the previous problem) for the Universe consisting of non-relativistic matter and radiation. Show that motion with $\dot{a}>0$ in such a potential can only be decelerating. | ||
<div class="NavFrame collapsed"> | <div class="NavFrame collapsed"> | ||
| Line 267: | Line 293: | ||
We see that $dV/dx>0$ for all $x$, therefore $\ddot{x}=-dV/dx<0$.</p> | We see that $dV/dx>0$ for all $x$, therefore $\ddot{x}=-dV/dx<0$.</p> | ||
</div> | </div> | ||
| − | </div> | + | </div></div> |
| + | |||
| + | |||
<div id="dyn39"></div> | <div id="dyn39"></div> | ||
| − | === Problem 12 | + | <div style="border: 1px solid #AAA; padding:5px;"> |
| + | === Problem 12: exact solutions with dust, radiation and curvature === | ||
Derive the exact solutions of the Friedman equations for the Universe with arbitrary curvature, filled with radiation and matter. | Derive the exact solutions of the Friedman equations for the Universe with arbitrary curvature, filled with radiation and matter. | ||
<div class="NavFrame collapsed"> | <div class="NavFrame collapsed"> | ||
| Line 277: | Line 306: | ||
<p style="text-align: left;">Following the consideration of the dynamics of the Universe in terms of an effective potential for variable $a=a/a_{0}$, for the Universe filled with dust ($w=0$) and radiation ($w=1/3$) we use the formulas (\ref{BBpotential1}),(\ref{BBpotential2}), and (\ref{BBpotential3}). Then the solution in quadratures is | <p style="text-align: left;">Following the consideration of the dynamics of the Universe in terms of an effective potential for variable $a=a/a_{0}$, for the Universe filled with dust ($w=0$) and radiation ($w=1/3$) we use the formulas (\ref{BBpotential1}),(\ref{BBpotential2}), and (\ref{BBpotential3}). Then the solution in quadratures is | ||
\[\tau=\int\limits_{0}^{x}\frac{x\,dx} | \[\tau=\int\limits_{0}^{x}\frac{x\,dx} | ||
| − | {\sqrt{\Omega_{k0}x^{2}+\Omega_{m0}x+\Omega_{r0}}} | + | {\sqrt{\Omega_{k0}x^{2}+\Omega_{m0}x+\Omega_{r0}}},\] |
| − | ,\] | + | |
which can be rewritten as | which can be rewritten as | ||
\[t=\sqrt{\frac{a_0}{H_0}}\;\int\limits_{0}^{a/a_0} | \[t=\sqrt{\frac{a_0}{H_0}}\;\int\limits_{0}^{a/a_0} | ||
| Line 284: | Line 312: | ||
where $\omega_{i}=8\pi G a_{0}^{2}\rho_{i0}$.</p> | where $\omega_{i}=8\pi G a_{0}^{2}\rho_{i0}$.</p> | ||
</div> | </div> | ||
| − | </div> | + | </div></div> |
| + | |||
| + | |||
| + | == Deceleration parameter == | ||
<div id="dyn41"></div> | <div id="dyn41"></div> | ||
| − | === Problem 13 | + | <div style="border: 1px solid #AAA; padding:5px;"> |
| + | === Problem 13: one component, flat case === | ||
Show that for a spatially flat Universe consisting of one component with equation of state $p = w\rho$ the deceleration parameter $q\equiv -\ddot{a}/(aH^2)$ is equal to $\frac{1}{2}(1 + 3w)$. | Show that for a spatially flat Universe consisting of one component with equation of state $p = w\rho$ the deceleration parameter $q\equiv -\ddot{a}/(aH^2)$ is equal to $\frac{1}{2}(1 + 3w)$. | ||
| + | <div class="NavFrame collapsed"> | ||
| + | <div class="NavHead">solution</div> | ||
| + | <div style="width:100%;" class="NavContent"> | ||
| + | <p style="text-align: left;">From the conservation equations | ||
| + | \[p = - \frac{\dot \rho }{3H}\frac{w}{1 + w}.\] | ||
| + | Then using that | ||
| + | \begin{align} | ||
| + | w& = \frac{2q - 1}{3}; \\ | ||
| + | \dot \rho &= \frac{3}{{4\pi G}}H\dot H,\quad \dot H = - H^2 (1 + q), | ||
| + | \end{align} | ||
| + | we find | ||
| + | \[p = \frac{H^2}{8\pi G}(2q -1)\] | ||
| + | </p> | ||
| + | </div> | ||
| + | </div></div> | ||
| + | |||
| + | |||
| + | |||
| + | <div id=""></div> | ||
| + | <div style="border: 1px solid #AAA; padding:5px;"> | ||
| + | === Problem 14: relation between the total pressure and the deceleration parameter === | ||
| + | Find the relation between the total pressure and the deceleration parameter for the flat Universe. | ||
<div class="NavFrame collapsed"> | <div class="NavFrame collapsed"> | ||
<div class="NavHead">solution</div> | <div class="NavHead">solution</div> | ||
| Line 299: | Line 353: | ||
\end{equation}</p> | \end{equation}</p> | ||
</div> | </div> | ||
| − | </div> | + | </div></div> |
| + | |||
| + | |||
| + | |||
| + | <div id=""></div> | ||
| + | <div style="border: 1px solid #AAA; padding:5px;"> | ||
| + | === Problem 15: $\frac{d^k p}{dt^k }$ === | ||
| + | The expansion of pressure by the cosmic time is given by | ||
| + | \[p(t) = \sum\limits_{k = 0}^\infty {\left. {\frac{1}{k!}\frac{d^k p}{dt^k }} \right|} _{t = t_0 } (t - t_0 )^k. \] | ||
| + | Using cosmography parameters (see Chapter 2), evaluate the derivatives $\frac{d^k p}{dt^k }$ up to the fourth order | ||
| + | <div class="NavFrame collapsed"> | ||
| + | <div class="NavHead">solution</div> | ||
| + | <div style="width:100%;" class="NavContent"> | ||
| + | <p style="text-align: left;"> | ||
| + | \begin{align} | ||
| + | p& = \frac{H^2 }{8\pi G}(2q - 1);\\ | ||
| + | \frac{dp}{dt} &= \frac{H^3 }{4\pi G}(1 - j);\\ | ||
| + | \frac{d^2 p}{dt^2} &= \frac{H^4 }{4\pi G}\left( {j - 3q - s - 3} \right);\\ | ||
| + | \frac{d^3 p}{dt^3 } &= \frac{H^5 }{4\pi G}\left[ {2s + j - l + q\left( {21 - j} \right) + 6q^2 + 12} \right];\\ | ||
| + | \frac{d^4 p}{dt^4 } &= \frac{H^6 }{4\pi G}\big[ | ||
| + | j^2 + 3l - m - 144q - 81q^2 - 6q^3 -12j(2 + q) - 3s - 3qs - 60\big]. | ||
| + | \end{align} | ||
| + | </p> | ||
| + | </div> | ||
| + | </div></div> | ||
| + | |||
| + | |||
| + | |||
| + | |||
| + | <div id=""></div> | ||
| + | <div style="border: 1px solid #AAA; padding:5px;"> | ||
| + | === Problem 16: $w(t)$ === | ||
| + | Сonsider the universe filled with an ideal fluid with the time-dependent equation of state parameter $w(t)$, and find $\rho (t),H(t)$. | ||
| + | <div class="NavFrame collapsed"> | ||
| + | <div class="NavHead">solution</div> | ||
| + | <div style="width:100%;" class="NavContent"> | ||
| + | <p style="text-align: left;">Combination of conservation law and first Friedmann equation gives | ||
| + | \[\dot \rho + 2\sqrt {6\pi G} \left[ {1 + w(t)} \right]\rho ^{3/2} = 0\] | ||
| + | This equation can be integrated to yield | ||
| + | \begin{align} | ||
| + | \rho &= \frac{1}{6\pi G\left[ {\int {dt\left( {1 + w(t)} \right)} } \right]^2 }, \\ | ||
| + | H(t) &= \frac{2}{3\int {dt\left( {1 + w(t)} \right)} } | ||
| + | \end{align} | ||
| + | If $w$ is a constant $\left( {w \ne - 1} \right)$, the standard expression for $\rho (t)$ and $H(t)$ is recovered. | ||
| + | </p> | ||
| + | </div> | ||
| + | </div></div> | ||
| + | |||
| + | |||
| + | |||
| + | |||
| + | <div id=""></div> | ||
| + | <div style="border: 1px solid #AAA; padding:5px;"> | ||
| + | === Problem 17: $\rho = f(a)$ === | ||
| + | Find the parameter $w$ in the equation of state if the dependence $\rho = f(a)$ is known. Check the obtained formula for the known special cases. | ||
| + | <div class="NavFrame collapsed"> | ||
| + | <div class="NavHead">solution</div> | ||
| + | <div style="width:100%;" class="NavContent"> | ||
| + | <p style="text-align: left;">The conservation equation | ||
| + | \[\dot \rho + 3H\rho (1 + w) = 0\] | ||
| + | can be rewritten in terms of $\rho = f(a)$ as | ||
| + | \[f'(a)\dot a + 3Hf(a)(1 + w) = 0,\] | ||
| + | whence we obtain | ||
| + | \[w = - \frac{1}{3}\frac{f'(a)}{f(a)}a - 1 = - \frac{1}{3}\frac{d\ln f(a)}{da}a - 1.\] | ||
| + | </p> | ||
| + | </div> | ||
| + | </div></div> | ||
| + | |||
| + | |||
<div id="dyn21_1"></div> | <div id="dyn21_1"></div> | ||
| − | === Problem | + | <div style="border: 1px solid #AAA; padding:5px;"> |
| − | Express the age of the Universe through the deceleration parameter $q=-\ddot{a}/(aH^2) | + | === Problem 18: age of the Universe === |
| + | Express the age of the spatially flat Universe filled with a single component with equation of state $p=w\rho$ through the deceleration parameter $q=-\ddot{a}/(aH^2)$. | ||
<div class="NavFrame collapsed"> | <div class="NavFrame collapsed"> | ||
<div class="NavHead">solution</div> | <div class="NavHead">solution</div> | ||
| Line 319: | Line 442: | ||
\quad t_{0}=\frac{2H_{0}^{-1}}{3(1+w)},\] | \quad t_{0}=\frac{2H_{0}^{-1}}{3(1+w)},\] | ||
thus $q$ is constant and | thus $q$ is constant and | ||
| − | \[t_{0}=\frac{ | + | \[t_{0}=\frac{H_{0}^{-1}}{1+q}.\]</p> |
</div> | </div> | ||
| − | </div> | + | </div></div> |
| + | |||
<div id="dyn41nn"></div> | <div id="dyn41nn"></div> | ||
| − | === Problem | + | <div style="border: 1px solid #AAA; padding:5px;"> |
| + | |||
| + | === Problem 19: non-flat generalization === | ||
Find the generalization of relation $q = \frac{1}{2}(1 + 3w)$ for the non-flat case. | Find the generalization of relation $q = \frac{1}{2}(1 + 3w)$ for the non-flat case. | ||
<div class="NavFrame collapsed"> | <div class="NavFrame collapsed"> | ||
| Line 341: | Line 467: | ||
\frac{\rho_{cr}}{\rho}\right)^{-1}.\]</p> | \frac{\rho_{cr}}{\rho}\right)^{-1}.\]</p> | ||
</div> | </div> | ||
| − | </div> | + | </div></div> |
| + | |||
<div id="dyn49nn"></div> | <div id="dyn49nn"></div> | ||
| − | === Problem | + | <div style="border: 1px solid #AAA; padding:5px;"> |
| + | === Problem 20: $q[\rho(a)]$ === | ||
Show that for a one-component Universe filled with ideal fluid of density $\rho$ | Show that for a one-component Universe filled with ideal fluid of density $\rho$ | ||
\[q=-1-\frac{1}{2}\,\frac{d\ln\rho}{d\ln a}.\] | \[q=-1-\frac{1}{2}\,\frac{d\ln\rho}{d\ln a}.\] | ||
| Line 356: | Line 484: | ||
\[q=-1-\frac{1}{2}\,\frac{d\ln\rho}{d\ln a}.\]</p> | \[q=-1-\frac{1}{2}\,\frac{d\ln\rho}{d\ln a}.\]</p> | ||
</div> | </div> | ||
| − | </div> | + | </div></div> |
| + | |||
<div id="dyn42"></div> | <div id="dyn42"></div> | ||
| − | === Problem | + | <div style="border: 1px solid #AAA; padding:5px;"> |
| + | === Problem 21: several components === | ||
Show that for a Universe consisting of several components with equations of state $p_{i} = w_{i} \rho_{i}$ the deceleration parameter is | Show that for a Universe consisting of several components with equations of state $p_{i} = w_{i} \rho_{i}$ the deceleration parameter is | ||
\[q = \frac{\Omega }{2} + | \[q = \frac{\Omega }{2} + | ||
| Line 380: | Line 510: | ||
\frac{3}{2}\sum\limits_i w_i\Omega _i.\]</p> | \frac{3}{2}\sum\limits_i w_i\Omega _i.\]</p> | ||
</div> | </div> | ||
| − | </div> | + | </div></div> |
| + | |||
<div id="dyn41n"></div> | <div id="dyn41n"></div> | ||
| − | === Problem | + | <div style="border: 1px solid #AAA; padding:5px;"> |
| − | For which values of state parameter $w$ the rate of expansion of a one-component flat Universe increases with time? | + | === Problem 22: acceleration or deceleration === |
| + | For which values of the state parameter $w$ the rate of expansion of a one-component flat Universe increases with time? | ||
<div class="NavFrame collapsed"> | <div class="NavFrame collapsed"> | ||
<div class="NavHead">solution</div> | <div class="NavHead">solution</div> | ||
| Line 393: | Line 525: | ||
w<-1.\]</p> | w<-1.\]</p> | ||
</div> | </div> | ||
| − | </div> | + | </div></div> |
| + | |||
| + | == Closed Universe == | ||
<div id="dyn34"></div> | <div id="dyn34"></div> | ||
| − | === Problem | + | <div style="border: 1px solid #AAA; padding:5px;"> |
| + | === Problem 23: closed dusty Universe, exact solution === | ||
Show that for a spatially closed ($k=1$) Universe that contains only non-relativistic matter the solution of the Friedman equations can be given in the form | Show that for a spatially closed ($k=1$) Universe that contains only non-relativistic matter the solution of the Friedman equations can be given in the form | ||
\[a(\eta)=a_{\star}(1-\cos\eta); | \[a(\eta)=a_{\star}(1-\cos\eta); | ||
| Line 432: | Line 567: | ||
\end{equation}</p> | \end{equation}</p> | ||
</div> | </div> | ||
| − | </div> | + | </div></div> |
| + | |||
<div id="dyn37"></div> | <div id="dyn37"></div> | ||
| − | === Problem | + | <div style="border: 1px solid #AAA; padding:5px;"> |
| + | === Problem 24: size and lifetime === | ||
Find the relation between the maximum size and the total lifetime of a closed Universe filled with dust. | Find the relation between the maximum size and the total lifetime of a closed Universe filled with dust. | ||
<div class="NavFrame collapsed"> | <div class="NavFrame collapsed"> | ||
| Line 443: | Line 580: | ||
\[T=\pi a_{\max}.\]</p> | \[T=\pi a_{\max}.\]</p> | ||
</div> | </div> | ||
| − | </div> | + | </div></div> |
| + | |||
<div id="dyn30"></div> | <div id="dyn30"></div> | ||
| − | === Problem | + | <div style="border: 1px solid #AAA; padding:5px;"> |
| + | === Problem 25: future through observables === | ||
Suppose we know the current values of the Hubble constant $H_0$ and the deceleration parameter $q_0$ for a closed Universe filled with dust only. How many times larger will it ever become? | Suppose we know the current values of the Hubble constant $H_0$ and the deceleration parameter $q_0$ for a closed Universe filled with dust only. How many times larger will it ever become? | ||
<div class="NavFrame collapsed"> | <div class="NavFrame collapsed"> | ||
| Line 476: | Line 615: | ||
\end{equation}</p> | \end{equation}</p> | ||
</div> | </div> | ||
| − | </div> | + | </div></div> |
| + | |||
<div id="dyn28"></div> | <div id="dyn28"></div> | ||
| − | === Problem | + | <div style="border: 1px solid #AAA; padding:5px;"> |
| + | === Problem 26: age of the Universe === | ||
In a closed Universe filled with non-relativistic matter the current values of the Hubble constant is $H_0$, the deceleration parameter is $q_0$. Find the current age of this Universe. | In a closed Universe filled with non-relativistic matter the current values of the Hubble constant is $H_0$, the deceleration parameter is $q_0$. Find the current age of this Universe. | ||
<div class="NavFrame collapsed"> | <div class="NavFrame collapsed"> | ||
| Line 494: | Line 635: | ||
\arccos(q_{0}^{-1}-1)\Big).\]</p> | \arccos(q_{0}^{-1}-1)\Big).\]</p> | ||
</div> | </div> | ||
| − | </div> | + | </div></div> |
| + | |||
<div id="dyn31"></div> | <div id="dyn31"></div> | ||
| − | === Problem | + | <div style="border: 1px solid #AAA; padding:5px;"> |
| + | === Problem 27: around the Universe === | ||
Suppose in the same Universe radiation is dominating during a negligibly small fraction of total time of evolution. How many times will a photon travel around the Universe during the time from its "birth" to its "death"? | Suppose in the same Universe radiation is dominating during a negligibly small fraction of total time of evolution. How many times will a photon travel around the Universe during the time from its "birth" to its "death"? | ||
<div class="NavFrame collapsed"> | <div class="NavFrame collapsed"> | ||
<div class="NavHead">solution</div> | <div class="NavHead">solution</div> | ||
<div style="width:100%;" class="NavContent"> | <div style="width:100%;" class="NavContent"> | ||
| − | <p style="text-align: left;">First of all, note, that in a stationary closed Universe a photon (as any other particle) that is emitted from a given point in arbitrary direction, travels "around the world" in finite time and returns to the initial point from the opposite direction, very much like a particle moving in a "straight line" on a two-dimensional sphere does. The trigonometric radial coordinate $\chi$, such that $d{\chi ^2} = d{r^2}/(1 - {r^2})$ (see problem | + | <p style="text-align: left;">First of all, note, that in a stationary closed Universe a photon (as any other particle) that is emitted from a given point in arbitrary direction, travels "around the world" in finite time and returns to the initial point from the opposite direction, very much like a particle moving in a "straight line" on a two-dimensional sphere does. The trigonometric radial coordinate $\chi$, such that $d{\chi ^2} = d{r^2}/(1 - {r^2})$ (see [[Friedman-Lemaitre-Robertson-Walker_(FLRW)_metric#equ20|problem of chapter 2]]), changes during the round-trip from $0$ to $\pi$ (on the opposite pole) and back to zero. |
In terms of conformal time $d\eta / a(t)$ and $\chi$ the interval for a radially propagating photon $(d\theta = d\varphi = 0)$ is | In terms of conformal time $d\eta / a(t)$ and $\chi$ the interval for a radially propagating photon $(d\theta = d\varphi = 0)$ is | ||
\[d{s^2} = a{(t)^2}(d{\eta ^2} - d{\chi ^2})=0,\] | \[d{s^2} = a{(t)^2}(d{\eta ^2} - d{\chi ^2})=0,\] | ||
| − | We know (see | + | We know (see [[#dyn34|problem]]) that the lifetime of the Universe, i.e. the interval between two zeros of $a(\eta)$ corresponds to the interval $\Delta\eta=2\pi$. During this time a photon will travel distance $\Delta\chi=2\pi$, thus making exactly one round-trip around the Universe.</p> |
</div> | </div> | ||
| − | </div> | + | </div></div> |
| + | |||
<div id="dyn57"></div> | <div id="dyn57"></div> | ||
| − | + | <div style="border: 1px solid #AAA; padding:5px;"> | |
| − | === Problem | + | === Problem 28: different volumes in dynamical Universe === |
In a closed Universe filled with dust the current value of the Hubble constant is $H_0$ and of the deceleration parameter $q_0$. | In a closed Universe filled with dust the current value of the Hubble constant is $H_0$ and of the deceleration parameter $q_0$. | ||
| Line 523: | Line 667: | ||
<div class="NavHead">solution</div> | <div class="NavHead">solution</div> | ||
<div style="width:100%;" class="NavContent"> | <div style="width:100%;" class="NavContent"> | ||
| − | <p style="text-align: left;">'''a)''' The volume of a closed Universe with scale factor $a_0$ was found in | + | <p style="text-align: left;">'''a)''' The volume of a closed Universe with scale factor $a_0$ was found in [[Friedman-Lemaitre-Robertson-Walker_(FLRW)_metric#equ22|problem]]: |
$V_{0}=2\pi^2 a_{0}^{3}$. | $V_{0}=2\pi^2 a_{0}^{3}$. | ||
| Line 545: | Line 689: | ||
\int\limits_{0}^{\eta_0}d\eta \sin^{2}\eta | \int\limits_{0}^{\eta_0}d\eta \sin^{2}\eta | ||
\end{align*} | \end{align*} | ||
| − | Assuming here $\eta_{0}=\pi$, which corresponds to the moment of maximal expansion, we obtain the total volume of the Universe calculated in [[#equ22|problem]] at this same moment. At later times $\eta>\pi$ we can see all of the Universe, moreover some points from different directions, so the formula is not applicable. | + | Assuming here $\eta_{0}=\pi$, which corresponds to the moment of maximal expansion, we obtain the total volume of the Universe calculated in [[Friedman-Lemaitre-Robertson-Walker_(FLRW)_metric#equ22|problem]] at this same moment. At later times $\eta>\pi$ we can see all of the Universe, moreover some points from different directions, so the formula is not applicable. |
'''c)''' The volume that we are observing directly differs from the calculated above as we see the distances between objects as they were at the moment of emission, not as they are "now", so one $a(t)$ shoulf be left inside the integral. Otherwise following the same steps, we arrive to | '''c)''' The volume that we are observing directly differs from the calculated above as we see the distances between objects as they were at the moment of emission, not as they are "now", so one $a(t)$ shoulf be left inside the integral. Otherwise following the same steps, we arrive to | ||
| Line 554: | Line 698: | ||
In more rigorous terms: consider the $4$-volume $\Omega$ filled with worldlines of all particles which we are currently observing (i.e. the ones the light from which took less time to arrive to us than the age of the Universe). The previous volume $V_{b}$ was the spatial volume that the observed matter occupies "at present", i.e. it is the volume of the section $t=t_{0}$ of $\Omega$. The volume $V_{c}$ is the 3-volume of the section of $\Omega$ by the light "cone" directed to the past with the vertex at the observer (it is actually a cone only in Minkowski spacetime, and is quite deformed for Friedmannian spaces).</p> | In more rigorous terms: consider the $4$-volume $\Omega$ filled with worldlines of all particles which we are currently observing (i.e. the ones the light from which took less time to arrive to us than the age of the Universe). The previous volume $V_{b}$ was the spatial volume that the observed matter occupies "at present", i.e. it is the volume of the section $t=t_{0}$ of $\Omega$. The volume $V_{c}$ is the 3-volume of the section of $\Omega$ by the light "cone" directed to the past with the vertex at the observer (it is actually a cone only in Minkowski spacetime, and is quite deformed for Friedmannian spaces).</p> | ||
</div> | </div> | ||
| − | </div> | + | </div></div> |
| + | |||
| + | == Different problems == | ||
<div id="dyn_open"></div> | <div id="dyn_open"></div> | ||
| − | === Problem | + | <div style="border: 1px solid #AAA; padding:5px;"> |
| + | === Problem 29: open dusty Universe === | ||
Find the solution of Friedman equations for spatially open ($k=-1$) Universe filled with dust in the parametric form $a(\eta)$, $t(\eta)$. | Find the solution of Friedman equations for spatially open ($k=-1$) Universe filled with dust in the parametric form $a(\eta)$, $t(\eta)$. | ||
<div class="NavFrame collapsed"> | <div class="NavFrame collapsed"> | ||
<div class="NavHead">solution</div> | <div class="NavHead">solution</div> | ||
<div style="width:100%;" class="NavContent"> | <div style="width:100%;" class="NavContent"> | ||
| − | <p style="text-align: left;">The solution differs from the one for $k=+1$ ( | + | <p style="text-align: left;">The solution differs from the one for $k=+1$ ([[#dyn34|problem]]) only by the sign of $k$. Likewise the first Friedman equation is rewritten as |
\[\frac 1{a^4}\left(\frac{da}{d\eta} \right)^2 | \[\frac 1{a^4}\left(\frac{da}{d\eta} \right)^2 | ||
=\frac{2a_{\star}}{a^3}+\frac{1}{a^2}, | =\frac{2a_{\star}}{a^3}+\frac{1}{a^2}, | ||
| Line 578: | Line 725: | ||
\end{align}</p> | \end{align}</p> | ||
</div> | </div> | ||
| − | </div> | + | </div></div> |
| + | |||
<div id="dyn40"></div> | <div id="dyn40"></div> | ||
| − | === Problem | + | <div style="border: 1px solid #AAA; padding:5px;"> |
| + | === Problem 30: density evolution === | ||
Suppose the density of some component in a spatially flat Universe depends on scale factor as $\rho(t) \sim a^{-n}(t)$. How much time is needed for the density of this component to change from $\rho_1$ to $\rho_2$? | Suppose the density of some component in a spatially flat Universe depends on scale factor as $\rho(t) \sim a^{-n}(t)$. How much time is needed for the density of this component to change from $\rho_1$ to $\rho_2$? | ||
<div class="NavFrame collapsed"> | <div class="NavFrame collapsed"> | ||
| Line 595: | Line 744: | ||
\big(\sqrt{\rho_1}-\sqrt{\rho_2}\big).\]</p> | \big(\sqrt{\rho_1}-\sqrt{\rho_2}\big).\]</p> | ||
</div> | </div> | ||
| − | </div> | + | </div></div> |
| + | |||
<div id="dyn44"></div> | <div id="dyn44"></div> | ||
| − | === Problem | + | <div style="border: 1px solid #AAA; padding:5px;"> |
| + | === Problem 31: $q[H(t)]$ === | ||
Using the expression for $H(t)$, calculate the deceleration parameter for the cases of domination of | Using the expression for $H(t)$, calculate the deceleration parameter for the cases of domination of | ||
| Line 619: | Line 770: | ||
'''b)''' for matter $q=1$.</p> | '''b)''' for matter $q=1$.</p> | ||
</div> | </div> | ||
| − | </div> | + | </div></div> |
| + | |||
<div id="dyn77"></div> | <div id="dyn77"></div> | ||
| − | === Problem | + | <div style="border: 1px solid #AAA; padding:5px;"> |
| + | === Problem 32: effective EoS for multi-component Universe === | ||
Consider a Universe consisting of $n$ components, with equations of state $p_{i}=w_{i}\rho_{i}$, and find $w_{tot}$, the parameter of the equation of state $p_{tot}=w_{tot}\rho_{tot}$. | Consider a Universe consisting of $n$ components, with equations of state $p_{i}=w_{i}\rho_{i}$, and find $w_{tot}$, the parameter of the equation of state $p_{tot}=w_{tot}\rho_{tot}$. | ||
<div class="NavFrame collapsed"> | <div class="NavFrame collapsed"> | ||
| Line 637: | Line 790: | ||
\end{equation}</p> | \end{equation}</p> | ||
</div> | </div> | ||
| − | </div> | + | </div></div> |
| + | |||
<div id="dyn78"></div> | <div id="dyn78"></div> | ||
| − | === Problem | + | <div style="border: 1px solid #AAA; padding:5px;"> |
| + | === Problem 33: relative densities === | ||
Derive the equations of motion for relative densities $\Omega_{i}=\rho_{i}/\rho_{cr}$ of the two components comprising a spatially flat two-component Universe, if their equations of state are $p=w_i\rho$, $i=1,2$. | Derive the equations of motion for relative densities $\Omega_{i}=\rho_{i}/\rho_{cr}$ of the two components comprising a spatially flat two-component Universe, if their equations of state are $p=w_i\rho$, $i=1,2$. | ||
<div class="NavFrame collapsed"> | <div class="NavFrame collapsed"> | ||
| Line 659: | Line 814: | ||
=3H\Omega_{m}\Omega_{r}(w_{r}-w_{m}).\]</p> | =3H\Omega_{m}\Omega_{r}(w_{r}-w_{m}).\]</p> | ||
</div> | </div> | ||
| − | </div> | + | </div></div> |
| + | |||
| + | |||
<div id="dyn60"></div> | <div id="dyn60"></div> | ||
| − | === Problem | + | <div style="border: 1px solid #AAA; padding:5px;"> |
| + | === Problem 34: EoS for non-relativistic gas === | ||
Suppose a Universe is initially filled with a gas of non-relativistic particles of mass density $\rho_{0}$, pressure $p_{0}$, and $c_p/c_v=\gamma$. Construct the equation of state for such a system. | Suppose a Universe is initially filled with a gas of non-relativistic particles of mass density $\rho_{0}$, pressure $p_{0}$, and $c_p/c_v=\gamma$. Construct the equation of state for such a system. | ||
<div class="NavFrame collapsed"> | <div class="NavFrame collapsed"> | ||
| Line 689: | Line 847: | ||
\left(\frac{p}{p_{0}}\right)^{1/\gamma}.\]</p> | \left(\frac{p}{p_{0}}\right)^{1/\gamma}.\]</p> | ||
</div> | </div> | ||
| − | </div> | + | </div></div> |
| + | |||
| + | |||
<div id="dyn19"></div> | <div id="dyn19"></div> | ||
| − | === Problem | + | <div style="border: 1px solid #AAA; padding:5px;"> |
| + | === Problem 35: Newtonian derivation of critical density === | ||
Derive the expression for the critical density $\rho_{cr}$ from the condition that Hubble's expansion velocity equals the second cosmic velocity (escape velocity) $v=\sqrt{2gR}$. | Derive the expression for the critical density $\rho_{cr}$ from the condition that Hubble's expansion velocity equals the second cosmic velocity (escape velocity) $v=\sqrt{2gR}$. | ||
<div class="NavFrame collapsed"> | <div class="NavFrame collapsed"> | ||
| Line 703: | Line 864: | ||
\[\rho_{cr}=\frac{3H^2}{8\pi G}.\]</p> | \[\rho_{cr}=\frac{3H^2}{8\pi G}.\]</p> | ||
</div> | </div> | ||
| − | </div> | + | </div></div> |
| + | |||
| + | == X-component == | ||
<div id="dyn42nn"></div> | <div id="dyn42nn"></div> | ||
| − | + | <div style="border: 1px solid #AAA; padding:5px;"> | |
| − | === Problem | + | === Problem 36: dust plus something else === |
Suppose the Universe is filled with non-relativistic matter and some substance with equation of state $p_X=w\rho_X$. Find the evolution equation for the quantity $r \equiv \frac{\rho_m}{\rho _X}$. | Suppose the Universe is filled with non-relativistic matter and some substance with equation of state $p_X=w\rho_X$. Find the evolution equation for the quantity $r \equiv \frac{\rho_m}{\rho _X}$. | ||
<div class="NavFrame collapsed"> | <div class="NavFrame collapsed"> | ||
| Line 713: | Line 876: | ||
<div style="width:100%;" class="NavContent"> | <div style="width:100%;" class="NavContent"> | ||
<p style="text-align: left;">From $r=\rho_{m}/\rho_{X}$ | <p style="text-align: left;">From $r=\rho_{m}/\rho_{X}$ | ||
| − | \[\dot r = \frac | + | \[\dot r = \frac{{\dot \rho}_m}{\rho _X} |
| − | - | + | - {\dot \rho }_X{\rho_m}{\rho _X^2}.\] |
Then on substitution of ${\rho _m}$ and ${\rho _X}$ from the corresponding conservation laws, we get | Then on substitution of ${\rho _m}$ and ${\rho _X}$ from the corresponding conservation laws, we get | ||
\[\dot r = 3Hwr.\]</p> | \[\dot r = 3Hwr.\]</p> | ||
</div> | </div> | ||
| − | </div> | + | </div></div> |
| + | |||
<div id="dyn43nn"></div> | <div id="dyn43nn"></div> | ||
| − | === Problem | + | <div style="border: 1px solid #AAA; padding:5px;"> |
| + | === Problem 37: deceleration parameter === | ||
Express the deceleration parameter through the ratio $r$ for the conditions of the previous problem. | Express the deceleration parameter through the ratio $r$ for the conditions of the previous problem. | ||
<div class="NavFrame collapsed"> | <div class="NavFrame collapsed"> | ||
<div class="NavHead">solution</div> | <div class="NavHead">solution</div> | ||
<div style="width:100%;" class="NavContent"> | <div style="width:100%;" class="NavContent"> | ||
| − | <p style="text-align: left;">Similarly to problem | + | <p style="text-align: left;">Similarly to [[#dyn41|problem]], on substitution of the right hand parts of the Friedman equations into the numerator and denominator, we get |
| − | \[q = - \frac{\ddot{a}/a}{H^2} | + | \[q = - \frac{\ddot{a}/a}{H^2} = \frac{\frac{4\pi G}{3}(\rho + 3p)}{\frac{8\pi G}{3}\rho } |
| − | + | = \frac{1}{2}\frac{\rho_m + \rho_X + 3w\rho _X}{\rho_m +\rho _X} | |
| − | + | ||
| − | = \frac{1}{2}\frac{\rho_m + \rho_X + 3w\rho _X} | + | |
| − | + | ||
=\frac{1}{2} + \frac{3w}{2(1+r)}.\] | =\frac{1}{2} + \frac{3w}{2(1+r)}.\] | ||
Another way: | Another way: | ||
| − | \[q = - 1 + \frac{d} | + | \[q = - 1 + \frac{d}{dt}\left( \frac{1}{H} \right) |
| − | = - 1 - \frac | + | = - 1 - \frac{\dot H}{H^2};\] |
\[\dot H = - 4\pi G(\rho + p), | \[\dot H = - 4\pi G(\rho + p), | ||
| − | \quad {H^2} = \frac | + | \quad {H^2} = \frac{8\pi G}{3}\rho.\]</p> |
</div> | </div> | ||
| − | </div> | + | </div></div> |
| + | |||
<div id="dyn46nn"></div> | <div id="dyn46nn"></div> | ||
| − | === Problem | + | <div style="border: 1px solid #AAA; padding:5px;"> |
| + | === Problem 38: $\rho_{X}\propto H^2$ === | ||
Let a spatially flat Universe be filled with non-relativistic dust and a substance with equation of state $p_{X}= w\rho_{X}$. Show that in case $\rho_{X}\propto H^2$, the ratio $r =\rho_{m}/\rho_{X}$ does not depend on time. | Let a spatially flat Universe be filled with non-relativistic dust and a substance with equation of state $p_{X}= w\rho_{X}$. Show that in case $\rho_{X}\propto H^2$, the ratio $r =\rho_{m}/\rho_{X}$ does not depend on time. | ||
<div class="NavFrame collapsed"> | <div class="NavFrame collapsed"> | ||
| Line 748: | Line 912: | ||
<div style="width:100%;" class="NavContent"> | <div style="width:100%;" class="NavContent"> | ||
<p style="text-align: left;">The Friedman equations can be written as | <p style="text-align: left;">The Friedman equations can be written as | ||
| − | \[3{H^2} = 8\pi \rho - 3\frac{k} | + | \[3{H^2} = 8\pi \rho - 3\frac{k}{a^2},\] |
\[\dot H | \[\dot H | ||
| − | = - 4\pi G\left( {\rho + p} \right) + \frac{k} | + | = - 4\pi G\left( {\rho + p} \right) + \frac{k}{a^2}.\] |
Then | Then | ||
\[2\frac{{\dot{H}}}{H} | \[2\frac{{\dot{H}}}{H} | ||
| Line 756: | Line 920: | ||
-\frac{k}{{{a}^{2}}H}\left( 1+3\frac{p}{\rho } \right).\] | -\frac{k}{{{a}^{2}}H}\left( 1+3\frac{p}{\rho } \right).\] | ||
Combining this with the conservation equation for $\rho_X$, we get | Combining this with the conservation equation for $\rho_X$, we get | ||
| − | \[\frac | + | \[\frac{\dot{\rho}_X}{\rho_X} |
| − | + | -2\frac{\dot{H}}{H} | |
=-\frac{3Hwr}{1+r}+\frac{k}{{{a}^{2}}H} | =-\frac{3Hwr}{1+r}+\frac{k}{{{a}^{2}}H} | ||
\left( 1+3\frac{w}{1+r} \right)\] | \left( 1+3\frac{w}{1+r} \right)\] | ||
| − | In the considered case (see | + | In the considered case (see [[#dyn42nn|problem]]) $\dot{r}=3Hwr$, so we arrive to |
\[\dot{r}=-\left( 1+r \right)\left[ \frac{{{{\dot{\rho }}}_{X}}}{{{\rho }_{X}}}-2\frac{{\dot{H}}}{H}-\frac{k}{{{a}^{2}}H}\left( 1+3\frac{w}{1+r} \right) \right].\] | \[\dot{r}=-\left( 1+r \right)\left[ \frac{{{{\dot{\rho }}}_{X}}}{{{\rho }_{X}}}-2\frac{{\dot{H}}}{H}-\frac{k}{{{a}^{2}}H}\left( 1+3\frac{w}{1+r} \right) \right].\] | ||
If ${{\rho }_{X}}\propto {{H}^{2}}$, then | If ${{\rho }_{X}}\propto {{H}^{2}}$, then | ||
| Line 767: | Line 931: | ||
Thus we obtain immediately that for a flat Universe $\dot{r}=0$.</p> | Thus we obtain immediately that for a flat Universe $\dot{r}=0$.</p> | ||
</div> | </div> | ||
| − | </div> | + | </div></div> |
| + | |||
<div id="dyn47nn"></div> | <div id="dyn47nn"></div> | ||
| − | === Problem | + | <div style="border: 1px solid #AAA; padding:5px;"> |
| + | === Problem 39: $r(q)$ === | ||
Show that for the model of the Universe described in the previous problem the parameter $r$ is related with the deceleration parameter as | Show that for the model of the Universe described in the previous problem the parameter $r$ is related with the deceleration parameter as | ||
\[\dot{r}=-2H\frac{\Omega_{curv}}{\Omega_X}q.\] | \[\dot{r}=-2H\frac{\Omega_{curv}}{\Omega_X}q.\] | ||
| Line 790: | Line 956: | ||
Yet again we confirm that for the considered model in the flat case $\dot{r}=0$.</p> | Yet again we confirm that for the considered model in the flat case $\dot{r}=0$.</p> | ||
</div> | </div> | ||
| − | </div> | + | </div></div> |
| + | |||
<div id="dyn48nn"></div> | <div id="dyn48nn"></div> | ||
| − | === Problem | + | <div style="border: 1px solid #AAA; padding:5px;"> |
| + | === Problem 40: $r$ in open and closed cases === | ||
Show that in the model of the Universe of [[#dyn46nn|problem]], in case $k=+1$ and $q>0$ (decelerated expansion) $r$ increases with time, in case $k=+1$ and $q<0$ (accelerated expansion) $r$ decreases with time, and for $k=-1$ vice-versa. | Show that in the model of the Universe of [[#dyn46nn|problem]], in case $k=+1$ and $q>0$ (decelerated expansion) $r$ increases with time, in case $k=+1$ and $q<0$ (accelerated expansion) $r$ decreases with time, and for $k=-1$ vice-versa. | ||
<div class="NavFrame collapsed"> | <div class="NavFrame collapsed"> | ||
| Line 801: | Line 969: | ||
{{{\Omega }_{X}}}q\] | {{{\Omega }_{X}}}q\] | ||
and definition | and definition | ||
| − | \[ | + | \[{\Omega }_{curv}=-\frac{k}{{{a}^{2}}{{H}^{2}}}.\]</p> |
</div> | </div> | ||
| − | </div> | + | </div></div> |
| − | + | ||
| − | + | ||
| − | '''The following | + | == Power-law cosmology == |
| + | '''The following problems on power-law cosmology are inspired by Kumar''' [http://arxiv.org/abs/1109.6924]. | ||
<div id="dyn-Kumar2"></div> | <div id="dyn-Kumar2"></div> | ||
| − | === Problem | + | <div style="border: 1px solid #AAA; padding:5px;"> |
| + | === Problem 41: power-law cosmologies === | ||
Let us consider a general class of power-law cosmologies described by the scale factor | Let us consider a general class of power-law cosmologies described by the scale factor | ||
\[a(t) =a_{0}\Big(\frac{t}{t_0}\Big)^\alpha,\] | \[a(t) =a_{0}\Big(\frac{t}{t_0}\Big)^\alpha,\] | ||
| Line 831: | Line 999: | ||
\[\alpha=\frac{1}{1+q},\qquad H=\frac{1}{(1+q)\;t}.\]</p> | \[\alpha=\frac{1}{1+q},\qquad H=\frac{1}{(1+q)\;t}.\]</p> | ||
</div> | </div> | ||
| − | </div> | + | </div></div> |
| + | |||
<div id="dyn-Kumar3"></div> | <div id="dyn-Kumar3"></div> | ||
| − | === Problem | + | <div style="border: 1px solid #AAA; padding:5px;"> |
| + | === Problem 42: age of the Universe === | ||
In the power-law cosmology find the age of the Universe at redshift $z$. | In the power-law cosmology find the age of the Universe at redshift $z$. | ||
<div class="NavFrame collapsed"> | <div class="NavFrame collapsed"> | ||
| Line 842: | Line 1,012: | ||
\[t=\int dt=\int \frac{da/a}{H} | \[t=\int dt=\int \frac{da/a}{H} | ||
=\int\limits_{z}^{\infty} \frac{dz}{(1+z)H}.\] | =\int\limits_{z}^{\infty} \frac{dz}{(1+z)H}.\] | ||
| − | Then using the result of the previous | + | Then using the result of the previous [[#dyn-Kumar2|problem]]), on integration we find |
\[t(z)=\frac{\alpha/H_{0}}{(1+z)^{1/\alpha}}.\]</p> | \[t(z)=\frac{\alpha/H_{0}}{(1+z)^{1/\alpha}}.\]</p> | ||
</div> | </div> | ||
| − | </div> | + | </div></div> |
| + | |||
<div id="dyn-Kumar4"></div> | <div id="dyn-Kumar4"></div> | ||
| − | === Problem | + | <div style="border: 1px solid #AAA; padding:5px;"> |
| + | === Problem 43: luminosity distance === | ||
For the power-law cosmology find the luminosity distance between the observer and the object with redshift $z$. | For the power-law cosmology find the luminosity distance between the observer and the object with redshift $z$. | ||
<div class="NavFrame collapsed"> | <div class="NavFrame collapsed"> | ||
| Line 860: | Line 1,032: | ||
(1+z)\big[1-(1+z)^{1-q}\big].\]</p> | (1+z)\big[1-(1+z)^{1-q}\big].\]</p> | ||
</div> | </div> | ||
| − | </div> | + | </div></div> |
| + | |||
| + | |||
| + | |||
| + | |||
| + | <div id=""></div> | ||
| + | <div style="border: 1px solid #AAA; padding:5px;"> | ||
| + | === Problem 44: a "softer" version of the cosmological evolution === | ||
| + | The power law cosmological evolution $a(t)\propto t^{\beta }$ leads to the Hubble parameter $H(t)\propto 1/t$. Consider a "softer" version of the cosmological evolution given by the law | ||
| + | \[H(t)=\frac{S}{t^{\alpha } } \] | ||
| + | where $S$ is a positive constant and $0<\alpha <1$. Analyze the dynamics of such model at $t\to 0$. | ||
| + | <br/> | ||
| + | ([http://arxiv.org/abs/0801.2348 F.Cannata, A.Kamenshchik, D.Regoli, arXiv:0801.2348]). | ||
| + | <div class="NavFrame collapsed"> | ||
| + | <div class="NavHead">solution</div> | ||
| + | <div style="width:100%;" class="NavContent"> | ||
| + | <p style="text-align: left;">Integrating $\frac{d}{dt} \left(\ln a\right)=\frac{S}{t^{\alpha } } $ we obtain | ||
| + | \[\ln \left(\frac{a(t)}{a(0)} \right)=\frac{S}{1-\alpha } t^{1-\alpha } \] | ||
| + | At $t=0$ singularity is present, but it is different from the traditional Big Bang singularity. If $t\ne 0\, (t>0)$, the right hand side is finite and hence one cannot have $a\left(0\right)=0$ in the left hand side. Then $a(0)>0$, while | ||
| + | \[\dot{a}(t)=a(0)\frac{S}{t^{\alpha } } \exp \left(\frac{S}{1-\alpha } t^{1-\alpha } \right)\mathop{\to }\limits_{t\to 0} \, \infty. \] | ||
| + | This type of singularity received the name of "soft Big Bang singularity" because the cosmological scale factor is finite (and non-zero) while its time derivative, the Hubble variable and the scalar curvature are singular. It is interesting to note that when $t\to \infty $ both $a(t)$ and $\dot{a}(t)$ tend to infinity, but they do not encounter any cosmological singularity because the Hubble variable and its derivatives tend to zero. | ||
| + | </p> | ||
| + | </div> | ||
| + | </div></div> | ||
Latest revision as of 11:03, 5 August 2014
Contents
- 1 One component dominated Universe
- 2 Matter and radiation
- 3 Non-interacting components, effective potential
- 4 Deceleration parameter
- 4.1 Problem 13: one component, flat case
- 4.2 Problem 14: relation between the total pressure and the deceleration parameter
- 4.3 Problem 15: $\frac{d^k p}{dt^k }$
- 4.4 Problem 16: $w(t)$
- 4.5 Problem 17: $\rho = f(a)$
- 4.6 Problem 18: age of the Universe
- 4.7 Problem 19: non-flat generalization
- 4.8 Problem 20: $q[\rho(a)]$
- 4.9 Problem 21: several components
- 4.10 Problem 22: acceleration or deceleration
- 5 Closed Universe
- 6 Different problems
- 7 X-component
- 8 Power-law cosmology
One component dominated Universe
Problem 1: matter or radiation in a flat Universe
Derive $\rho(a)$, $\rho(t)$ and $ a(t)$ for a spatially flat$^*$ ($k=0$) Universe that consists of only
a) radiation,
b) non-relativistic matter$^{**}$.
$^*$ The "spatial" part is often omitted when there cannot be any confusion (and even when there can be), generally and hereatfer.
$^{**}$ In this context and below also quite often called just "matter" or dust.
a) Radiation: $p=\frac{1}{3}\rho$, and from energy conservation \[\dot{\rho}+4\frac{\dot{a}}{a}\rho=0, \quad\Rightarrow\quad \rho\sim \frac{1}{a^{4}}.\] On substitution of this into the first Friedman equation we obtain the equation for $a(t)$ (we use normalization $a_{0}=1$) \[\frac{\dot{a}^{2}}{a^{2}} =\frac{8\pi G}{3}\cdot\frac{\rho_{r0}}{a^4}.\] After integration \[a(t) = \sqrt[4]{\tfrac{32}{3} \pi G{\rho _{r0}}}\;t^{1/2} \quad\mbox{and}\quad \rho_r (t)= \frac 1{\frac {32}{3}\pi G{t^2}}.\] The main result is: \begin{equation}\label{dyn-radiation} \rho_{r}\sim \frac{1}{a^{4}}, \quad a(t) \sim t^{1/2}, \quad \rho_{r}(t)\sim t^{-2}. \end{equation} b) For non-relativistic matter $p=0$, so the energy conservation equation is \[\dot{\rho}+3\frac{\dot{a}}{a}\rho=0, \quad\Rightarrow\quad \rho\sim \frac{1}{a^{3}}.\] In the same way as for the case of radiation domination we get \[a(t) = \sqrt[3]{{6\pi G{\rho _{m0}}}}\;t^{\frac{2}{3}} \quad\mbox{and}\quad \rho_m (t) = \frac{1}{6\pi Gt^2}.\] Then \begin{equation}\label{dyn-matter} \rho_{m}\sim\frac{1}{a^3}, \quad a(t) \sim t^{\frac{2}{3}}, \quad \rho_m (t) \sim t^{-2 }. \end{equation} Note that the dependence $\rho(t)$ is the same in both cases.
Problem 2: RD and MD regimes
Consider two spatially flat Universes. One is filled with radiation (thus radiation dominated, or RD), the other with dust (thus matter dominated, MD). The current energy density is the same. Compare energy densities when both of them are twice as old.
The temporal dependence of energy density in a Universe filled with only one component with equation of state $p=w\rho$ (where $w=const$) can be written as \[\rho(t)=\rho_{0}\frac{t_0^2}{t^2},\] where $\rho_0$ is the current density and \[t_0=\frac{1}{(w+1)\sqrt{6\pi G\rho_0}}\] is the current age of the Universe. When both Universes are twice older, density will be four times smaller in each of them.
Problem 3: RD$\to$MD transition
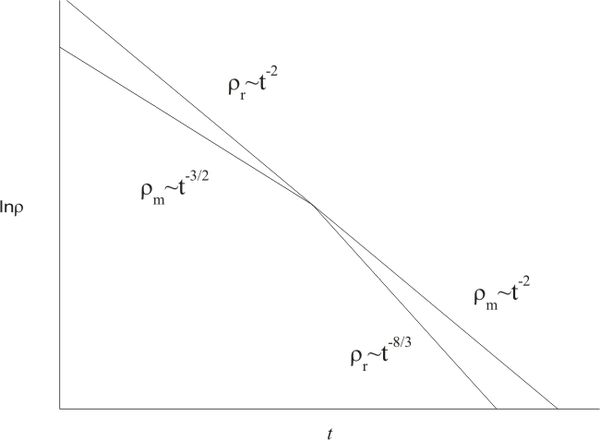
Find the scale factor and density of each component as functions of time in a flat Universe which consists of dust and radiation, for the case one of the components is dominating. Present the results graphically.
If matter and radiation do not interact, as is assumed, we can write down energy conservation laws for them separately. Then for radiation $\rho_{r}\sim a^{-4}$ and for matter $\rho_{m}\sim a^{-3}$, so energy density of radiation in an expanding Universe always decreases faster than that of non-relativistic matter. Thus the dynamics of the Universe can be divided into two stages: the first one when the dominating component is radiation, and the second one when the dominating component is non-relativistic matter.
The first stage: radiation domination. The first Friedman equation is
\[\left(\frac{\dot a}{a}\right)^2
= \frac{8\pi G}{3}(\rho_r + \rho_m)\]
When $\rho_r \gg \rho_m$, it takes the form
\[\left( \frac{\dot a}{a} \right)^2
\approx\frac{8\pi G}{3}\rho_r.\]
Then $a(t)\sim t^{1/2}$ and $\rho_r \sim t^{-2}.$
The dominating component determines the behavior of scale factor as function of time. Therefore we have to plug $a(t)$ for the Universe with dominating radiation into $\rho_m = \rho_m(a)$. Then $\rho_m \sim a^{-3} \sim t^{3/2}$. The relative density of matter increases with time, so the stage of radiation domination is succeeded by the stage of matter domination.
Stage 2: matter domination. Following the same train of thought we get
\[ a \sim t^{2/3};\quad\rho _m \sim \frac{1}{t^2};
\quad\rho _r \sim \frac{1}{a^4}
\sim \frac{1}{t^{8/3}}.\]
Problem 4: Hubble parameter
Derive the time dependence of the Hubble parameter for a flat Universe in which either matter or radiation is dominating.
a) matter domination
$H \equiv\dot a/a = 2/(3t).$
b) radiation domination
$H =1/(2t).$
Matter and radiation
Problem 5: exact solutions for matter and radiation
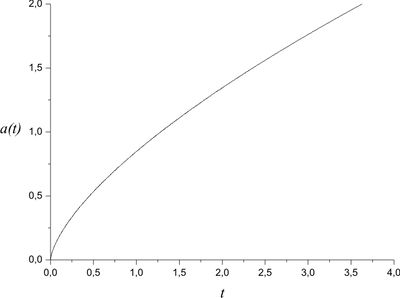
Derive the exact solutions of the Friedman equations for the Universe filled with matter and radiation. Plot the graphs of scale factor and both densities as functions of time.
Let us present the total energy density as the sum $\rho=\rho_m+\rho _r$ and plug it into the first Friedman equation \[\left( \frac{\dot a}{a} \right)^2 = \frac{8\pi G}{3}(\rho _r + \rho _m).\] The components' densities $\rho _m$ and $\rho _r$, according to problem, are \[\rho_m=\rho_{m0}\left(\frac{a_0}{a}\right)^{3},\quad \rho _r =\rho_{r0}\left(\frac{a_0}{a}\right)^{4}.\] By definition, $\Omega _i = \rho _i/{\rho _{cr}}$, where \[\rho _{cr} \equiv \frac{3H_0^2}{8\pi G}.\] Then \[\frac{\dot a}{a}= H_0\sqrt{\Omega_{m0}\left(\frac{a_0}{a} \right)^{3} +\Omega _{r0}\left(\frac{a_0}{a} \right)^{4}},\] therefore \begin{equation}\label{dyn-MRgenSol} t = \frac{1}{H_0}\int\limits_{0}^{a/a_0} \frac{dx}{x\sqrt{\Omega_{m0}x^{-3} +\Omega_{r0}x^{ - 4}}} =\frac{1}{H_0}\int\limits_{0}^{a/a_0} \frac{x\,dx}{\sqrt{\Omega_{m0}x +\Omega_{r0}}}. \end{equation}
Problem 6: equipartition time
At what moment after the Big Bang did matter's density exceed that of radiation for the first time?
The condition which determines the time $t_{md}$ when radiation and matter densities became equal is, obviously, $\rho_m=\rho_r$. As $\rho_{m}\sim a^{-3}$ and $\rho_{r}\sim a^{-4}$, then (see problem) \[a_{md} = \frac{\Omega _{r0}}{\Omega _{m0}}a_0.\] Using the results and notation of problem, we get \[t_{md} = \frac{1}{H_0}\int\limits_0^{a_{md}/a_{0}} \frac{x\,dx}{\sqrt {\Omega_{m0}x+ \Omega_{r0}}} =H_{0}^{-1} \frac{\Omega_{r0}^{3/2}}{\Omega_{m0}^{2}} \int\limits_{0}^{1}\frac{y\,dy}{\sqrt{y+1}}=\frac{2(2-\sqrt{2})}{3H_0} \frac{\Omega _{r0}^{3/2}}{\Omega _{m0}^2}.\]
Problem 7: age of the Universe
Determine the age of the Universe in which either matter or radiation has always been dominating.
For the case of matter domination $a\sim t^{2/3}$ (see problem and problem), so $H=\dot{a}/a=2/(3t)$ and \[t_{0}=\frac{2}{3H_{0}}.\] For the case of radiation domination $a\sim t^{1/2}$, $H=\dot{a}/a=1/(2t)$ and \[t_{0}=\frac{1}{2H_{0}}\] Using the observed value $H_0 \approx 7.15\cdot 10^{ - 11}\mbox{yr}^{-1}$, we get $t_0 \approx 9.3 \cdot 10^{9}\mbox{yr}$ for matter and $t_0 \approx 7 \cdot 10^{9}\mbox{yr}$ for radiation.
Non-interacting components, effective potential
Problem 8: constant EoS parameter
Derive the dependence $a(t)$ for a spatially flat Universe that consists of matter with equation of state $p=w\rho$, assuming that the parameter $w$ does not change throughout the evolution.
The energy conservation equation is \[\dot \rho +3 \frac{\dot a}a(1+w)\rho=0.\] On separation of variables and integration with the initial condition $\rho(1)=\rho_0$ (assuming $a_0=1$), we get \begin{equation}\label{dyn-rho(a-w)} \rho(a)=\rho_0a^{-3(1+w)}. \end{equation} Plugging the obtained $\rho(a)$ into the first Friedman equation, we have \[\left( \frac{\dot a}{a} \right)^2 = \frac{8}{3}\pi G\rho_0a^{ - 3(1 + w)}\] and therefore \[a(t) = \left[ \frac{2t}{3(1 + w)} \sqrt {\frac{8}{3}\pi G{\rho _0}} \right]^{\frac{2}{3(1 + w)}},\] thus \begin{equation}\label{dyn-a(t-w)} a(t)=A_{0} t^{\frac{2}{3(1+w)}}. \end{equation}
Problem 9: $H(t)$
Find the Hubble parameter as function of time for the previous problem.
Using the result of the previous problem (\ref{dyn-a(t-w)}) and the definition of the Hubble parameter, we get \[H = \frac{\dot a}{a}= \frac{2}{3\left( {1 + w} \right)t}.\]
Problem 10: effective potential
Using the first Friedman equation, construct the effective potential $V(a)$, which governs the one-dimensional motion of a fictitious particle with coordinate $a(t)$, for the Universe filled with several non-interacting components.
The dynamics of the Universe filled with different components with energy densities $\rho_i$ and equations of state $p_i=w_i\rho_i$ is described by equations \begin{align*} \left( \frac{\dot a}{a} \right)^2 &= \frac{8\pi G}{3} \sum\limits_i \rho _i - \frac{k}{a^2 },\\ \frac{\ddot a}{a} &= - \frac{4\pi G}{3} \sum\limits_i \left(\rho _i + 3p_i \right). \end{align*} Let us use dimensionless variables \[x \equiv \frac{a}{a_0}; \quad \tau \equiv t\left| {H_0 } \right|; \quad \Omega _{i0} \equiv \frac{\rho _{i0}}{\rho _{cr0}}; \quad \rho _{cr0} \equiv \frac{3H_0^2}{8\pi G}; \quad \Omega _{k0}\equiv-\frac{k}{a_0^2 H_0^2}.\] It is assumed that $H_0 \neq 0$. The Friedman equations in terms of new variables are (the dot now denotes derivative by $\tau$) \begin{align*} \frac{1}{2}\dot{x}^2 &=+\frac{1}{2}\sum\limits_i \Omega _{i0} x^{-(1+3w_i)}+\frac{1}{2}\Omega _{k0};\\ \ddot x &=-\frac{1}{2}\sum\limits_i \Omega _{i0} (1 + 3w_i )x^{ - (2 + 3w_i )}. \end{align*} They can be rewritten as \begin{equation}\label{BBpotential1} \frac{1}{2}\dot x^2 + V(x) = E;\qquad \ddot x = - \frac{dV}{dx}, \end{equation} where \begin{equation}\label{BBpotential2} E = \frac{1}{2}\Omega _{k0} ;\qquad V(x) = - \frac{1}{2}\sum\limits_i \Omega _{i0} x^{ - (1 + 3w_i )}. \end{equation}
Problem 11: effective potential for radiation and dust
Construct the effective one-dimensional potential $V(a)$ (using the notation of the previous problem) for the Universe consisting of non-relativistic matter and radiation. Show that motion with $\dot{a}>0$ in such a potential can only be decelerating.
For matter $w_{m}=0$, for radiation $w_{r}=1/3$: \begin{equation}\label{BBpotential3} V(x) = - \frac{\Omega _{m0}}{2x} - \frac{\Omega _{r0}}{2x^2}. \end{equation} We see that $dV/dx>0$ for all $x$, therefore $\ddot{x}=-dV/dx<0$.
Problem 12: exact solutions with dust, radiation and curvature
Derive the exact solutions of the Friedman equations for the Universe with arbitrary curvature, filled with radiation and matter.
Following the consideration of the dynamics of the Universe in terms of an effective potential for variable $a=a/a_{0}$, for the Universe filled with dust ($w=0$) and radiation ($w=1/3$) we use the formulas (\ref{BBpotential1}),(\ref{BBpotential2}), and (\ref{BBpotential3}). Then the solution in quadratures is \[\tau=\int\limits_{0}^{x}\frac{x\,dx} {\sqrt{\Omega_{k0}x^{2}+\Omega_{m0}x+\Omega_{r0}}},\] which can be rewritten as \[t=\sqrt{\frac{a_0}{H_0}}\;\int\limits_{0}^{a/a_0} \frac{x\,dx}{\sqrt{-kx^2+\omega_{m}x+\omega_{r}}},\] where $\omega_{i}=8\pi G a_{0}^{2}\rho_{i0}$.
Deceleration parameter
Problem 13: one component, flat case
Show that for a spatially flat Universe consisting of one component with equation of state $p = w\rho$ the deceleration parameter $q\equiv -\ddot{a}/(aH^2)$ is equal to $\frac{1}{2}(1 + 3w)$.
From the conservation equations \[p = - \frac{\dot \rho }{3H}\frac{w}{1 + w}.\] Then using that \begin{align} w& = \frac{2q - 1}{3}; \\ \dot \rho &= \frac{3}[[:Template:4\pi G]]H\dot H,\quad \dot H = - H^2 (1 + q), \end{align} we find \[p = \frac{H^2}{8\pi G}(2q -1)\]
Problem 14: relation between the total pressure and the deceleration parameter
Find the relation between the total pressure and the deceleration parameter for the flat Universe.
Plugging the first and second Friedman equations into the numerator and denominator correspondingly, we obtain \begin{equation}\label{dyn-q(w)} q = - \frac{\ddot{a}/a}{H^2} = \frac{\frac{4\pi G}{3}\rho(1+3w)} {\frac{8\pi G}{3}\rho}= \frac{1 + 3w}{2}. \end{equation}
Problem 15: $\frac{d^k p}{dt^k }$
The expansion of pressure by the cosmic time is given by \[p(t) = \sum\limits_{k = 0}^\infty {\left. {\frac{1}{k!}\frac{d^k p}{dt^k }} \right|} _{t = t_0 } (t - t_0 )^k. \] Using cosmography parameters (see Chapter 2), evaluate the derivatives $\frac{d^k p}{dt^k }$ up to the fourth order
\begin{align} p& = \frac{H^2 }{8\pi G}(2q - 1);\\ \frac{dp}{dt} &= \frac{H^3 }{4\pi G}(1 - j);\\ \frac{d^2 p}{dt^2} &= \frac{H^4 }{4\pi G}\left( {j - 3q - s - 3} \right);\\ \frac{d^3 p}{dt^3 } &= \frac{H^5 }{4\pi G}\left[ {2s + j - l + q\left( {21 - j} \right) + 6q^2 + 12} \right];\\ \frac{d^4 p}{dt^4 } &= \frac{H^6 }{4\pi G}\big[ j^2 + 3l - m - 144q - 81q^2 - 6q^3 -12j(2 + q) - 3s - 3qs - 60\big]. \end{align}
Problem 16: $w(t)$
Сonsider the universe filled with an ideal fluid with the time-dependent equation of state parameter $w(t)$, and find $\rho (t),H(t)$.
Combination of conservation law and first Friedmann equation gives \[\dot \rho + 2\sqrt {6\pi G} \left[ {1 + w(t)} \right]\rho ^{3/2} = 0\] This equation can be integrated to yield \begin{align} \rho &= \frac{1}{6\pi G\left[ {\int {dt\left( {1 + w(t)} \right)} } \right]^2 }, \\ H(t) &= \frac{2}{3\int {dt\left( {1 + w(t)} \right)} } \end{align} If $w$ is a constant $\left( {w \ne - 1} \right)$, the standard expression for $\rho (t)$ and $H(t)$ is recovered.
Problem 17: $\rho = f(a)$
Find the parameter $w$ in the equation of state if the dependence $\rho = f(a)$ is known. Check the obtained formula for the known special cases.
The conservation equation \[\dot \rho + 3H\rho (1 + w) = 0\] can be rewritten in terms of $\rho = f(a)$ as \[f'(a)\dot a + 3Hf(a)(1 + w) = 0,\] whence we obtain \[w = - \frac{1}{3}\frac{f'(a)}{f(a)}a - 1 = - \frac{1}{3}\frac{d\ln f(a)}{da}a - 1.\]
Problem 18: age of the Universe
Express the age of the spatially flat Universe filled with a single component with equation of state $p=w\rho$ through the deceleration parameter $q=-\ddot{a}/(aH^2)$.
For a spatially flat one-component Universe with equation of state $p=w\rho$, where $w=const$, the scale factor depends on time as (\ref{dyn-a(t-w)}) \[ a(t)\sim t^{2/[3(1+w)]}.\] Then \begin{align*} H=\frac{\dot{a}}{a}&=\frac{2}{3(1+w)}\;\frac{1}{t},\\ \frac{\ddot{a}}{a}&=\frac{2}{3(1+w)} \Big(\frac{2}{3(1+w)}-1\Big)\frac{1}{t^{2}}, \end{align*} and we are immediately led to \[q=\frac{1+3w}{2}=const; \quad t_{0}=\frac{2H_{0}^{-1}}{3(1+w)},\] thus $q$ is constant and \[t_{0}=\frac{H_{0}^{-1}}{1+q}.\]
Problem 19: non-flat generalization
Find the generalization of relation $q = \frac{1}{2}(1 + 3w)$ for the non-flat case.
Excluding the combination $8\pi G$ from the Friedman equations, we get \[\frac{\ddot{a}}{a}= -\frac{1}{2}\left(H^{2}+\frac{k}{a^2}\right)(1+3w),\] therefore \[q = \frac{1+3w}{2} \left(1 + \frac{k}{a^{2}H^{2}}\right).\] We can derive another generalization by simply dividing one of the Friedman equations by the other: \[q=-\frac{\ddot{a}/a}{H^{2}}=\frac{1+3w}{2} \left(1-\frac{k}{a^{2}H^{2}} \frac{\rho_{cr}}{\rho}\right)^{-1}.\]
Problem 20: $q[\rho(a)]$
Show that for a one-component Universe filled with ideal fluid of density $\rho$ \[q=-1-\frac{1}{2}\,\frac{d\ln\rho}{d\ln a}.\]
The energy conservation law can be rewritten as \[a\,d\rho+3da(\rho+p)=0\quad\Leftrightarrow\quad \frac{a}{\rho}\frac{d\rho}{da}=-3(1+w).\] Then plugging $(1+w)$ into the formula for $q$ (\ref{dyn-q(w)}), we obtain \[q=-1-\frac{1}{2}\,\frac{d\ln\rho}{d\ln a}.\]
Problem 21: several components
Show that for a Universe consisting of several components with equations of state $p_{i} = w_{i} \rho_{i}$ the deceleration parameter is \[q = \frac{\Omega }{2} + \frac{3}{2}\sum\limits_i {w_i \Omega_i },\] where $\Omega$ is the total relative density.
Let us write the second Friedman equation for the multi-component model as \[\frac{\ddot a}{a} = \frac{4\pi G}{3}\sum\limits_i ( \rho _i + 3p_i).\] Plugging it into the definition of the deceleration parameter and using $H^2 = 8\pi G\rho_{cr}/3$, we get \[q = - \frac{\ddot aa}{\dot a^2} = \frac{\ddot{a}/a}{H^2} =\frac{\frac{4\pi G}{3}\sum\limits_i ( \rho _i + 3p_i)}{\frac{8\pi G}{3}\rho _{cr}}.\] Then \[q = \frac{\Omega }{2} + \frac{3}{2}\sum\limits_i w_i\Omega _i.\]
Problem 22: acceleration or deceleration
For which values of the state parameter $w$ the rate of expansion of a one-component flat Universe increases with time?
As $\dot{H}=-{H}^{2}(1+q)$, taking into account (\ref{dyn-q(w)}), we obtain \[\dot{H}<0\quad\Leftrightarrow\quad q=\frac{1+3w}{2}<-1\quad\Leftrightarrow\quad w<-1.\]
Closed Universe
Problem 23: closed dusty Universe, exact solution
Show that for a spatially closed ($k=1$) Universe that contains only non-relativistic matter the solution of the Friedman equations can be given in the form \[a(\eta)=a_{\star}(1-\cos\eta); \qquad t(\eta)=a_{\star}(\eta -\sin\eta); \qquad a_{\star}=\frac{4\pi G\rho_0}{3}; \quad 0<\eta<2\pi.\]
Let's use normalization of scale factor by the current value $a_{0}=1$. Taking into account that for matter $\rho\sim a^{-3}$, the first Friedman equation in terms of conformal time $d\eta=dt/a(t)$ is rewritten as \[\frac 1{a^4}\left(\frac{da}{d\eta} \right)^2 =\frac{2a_{\star}}{a^3}-\frac{1}{a^2},\] where \begin{equation}\label{aStar} a_{\star}=\frac{4\pi G \rho_{0}}{3}. \end{equation} After separation of variables and integration, one obtains \[\eta = \int\limits_{0}^{a/a_{\star}} \frac{dx}{\sqrt{2x-x^2}} =-\arccos \Big( 1 - \frac{a}{a_{\star}}\Big).\] Then \begin{align} \label{dyn-closed} a(\eta)&=a_{\star}(1-\cos\eta),\\ t(\eta)=\int a d\eta&=a_{\star}(\eta-\sin\eta), \qquad \eta\in(0,2\pi).\nonumber \end{align} Restoring $a_{0}$ by dimensionality, we get \begin{equation}\label{dyn-closed-dim} \frac{a}{a_0}=\alpha_{\star} (1-\cos\eta),\qquad \frac{t}{a_0}=\alpha_{\star} (\eta-\sin\eta),\qquad \alpha_{\star}=a_{0}^{2}a_{\star} =\frac{4\pi G}{3}\rho_{0}a_{0}^{2}. \end{equation}
Problem 24: size and lifetime
Find the relation between the maximum size and the total lifetime of a closed Universe filled with dust.
We make use of the exact solution in the form (\ref{dyn-closed}). As the range of values of $\eta$ is $[0,2\pi]$, the total lifetime is $T=2\pi a_{\star}$, while the maximal size is reached at $\eta=\pi$: $a_{\max}=2a_{\star}$. Then \[T=\pi a_{\max}.\]
Problem 25: future through observables
Suppose we know the current values of the Hubble constant $H_0$ and the deceleration parameter $q_0$ for a closed Universe filled with dust only. How many times larger will it ever become?
The current value of $a_0$ can be expressed through the observed quantities using the definition of the deceleration parameter $q$: \begin{equation}\label{dyn-a0} q=-\frac{\ddot{a}/a}{H^2} =\frac{\frac{4\pi G}{3}\rho}{H^2} =\frac{1}{2}\Big(1+\frac{1}{a^2 H^2}\Big), \quad\Rightarrow\quad a_0^2 H_0^2=\frac{1}{2q_0 - 1}. \end{equation} Then \[\alpha_{\star}=\frac{4\pi G }{3} \cdot \rho_0 a_{0}^{2} =\frac{a_{0}^{2}}{2} \Big(H_{0}^{2}+\frac{1}{a_{0}^{2}}\Big) =\frac{a_{0}^{2}H_{0}^{2}+1}{2} =\frac{q_0}{2q_0 -1}.\] The scale factor reaches its maximum at $\eta=\pi$ and \begin{equation}\label{dyn-alphaStar} \frac{a_{\max}}{a_0} =2\alpha_{\star}=\frac{1}{1-1/2q_{0}}. \end{equation} Then the maximal value of the size of the Universe can be written as \begin{equation}\label{dyn-Amax} a_{\max}=H_{0}^{-1}\frac{2q_{0}}{(2q_0 -1)^{3/2}}. \end{equation}
Problem 26: age of the Universe
In a closed Universe filled with non-relativistic matter the current values of the Hubble constant is $H_0$, the deceleration parameter is $q_0$. Find the current age of this Universe.
From the general solution (\ref{dyn-closed-dim}) \[t_{0}=a_{0}\alpha_{\star}(\eta_0 - \sin\eta_0),\] where, taking into account (\ref{dyn-alphaStar}), \[\eta_{0}=-\arccos(1-\alpha_\star^{-1}) =-\arccos(q_{0}^{-1}-1).\] On substitution of $a_0$ from (\ref{dyn-a0}), we get \[t_{0}=\frac{H_{0}^{-1}}{2q_0 -1}\Big( 1+\frac{q_0}{\sqrt{2q_0 -1}} \arccos(q_{0}^{-1}-1)\Big).\]
Problem 27: around the Universe
Suppose in the same Universe radiation is dominating during a negligibly small fraction of total time of evolution. How many times will a photon travel around the Universe during the time from its "birth" to its "death"?
First of all, note, that in a stationary closed Universe a photon (as any other particle) that is emitted from a given point in arbitrary direction, travels "around the world" in finite time and returns to the initial point from the opposite direction, very much like a particle moving in a "straight line" on a two-dimensional sphere does. The trigonometric radial coordinate $\chi$, such that $d{\chi ^2} = d{r^2}/(1 - {r^2})$ (see problem of chapter 2), changes during the round-trip from $0$ to $\pi$ (on the opposite pole) and back to zero. In terms of conformal time $d\eta / a(t)$ and $\chi$ the interval for a radially propagating photon $(d\theta = d\varphi = 0)$ is \[d{s^2} = a{(t)^2}(d{\eta ^2} - d{\chi ^2})=0,\] We know (see problem) that the lifetime of the Universe, i.e. the interval between two zeros of $a(\eta)$ corresponds to the interval $\Delta\eta=2\pi$. During this time a photon will travel distance $\Delta\chi=2\pi$, thus making exactly one round-trip around the Universe.
Problem 28: different volumes in dynamical Universe
In a closed Universe filled with dust the current value of the Hubble constant is $H_0$ and of the deceleration parameter $q_0$.
a) What is the total proper volume of the Universe at present time?
b) What is the total current proper volume of space occupied by matter which we are presently observing?
c) What is the total proper volume of space which we are directly observing?
a) The volume of a closed Universe with scale factor $a_0$ was found in problem: $V_{0}=2\pi^2 a_{0}^{3}$. Using the expression for $a_{0}$ through the measured quantities $H_0$ and $q_0$ (\ref{dyn-a0}), we get then \[V_0 = 2\pi^2a_0^3 = 2\pi^2 H_0^{-3}(2q_0-1)^{-3/2}.\] b) The spatial metric of the closed Universe is \[dl^{2}=a^{2}\big(d\chi^2 +\sin^{2}\!\chi\; d\Omega^{2}\big), \quad d\Omega^{2}=d\theta^2 +\sin^{2}\theta d\varphi^2,\] so the volume element between spherical layers of "radii" $\chi$ and $\chi+d\chi$ is \[dV_{\chi}=4\pi\cdot a^{2}\sin^{2}\chi \cdot a\,d\chi =4\pi a^{3}\sin^{2}\chi d\chi.\] At present we have received light emitted with the radial comoving coordinate $\chi$ equal to at most the conformal age of the Universe \[\eta_{0}=-\arccos(1-\alpha_{\star}^{-1}) =-\arccos(q_{0}^{-1}-1),\] where in the second equality sign we used (\ref{dyn-alphaStar}) . Then \begin{align*} V_{b}=4\pi a_{0}^{3} \int\limits_{0}^{\eta_0}d\eta \sin^{2}\eta \end{align*} Assuming here $\eta_{0}=\pi$, which corresponds to the moment of maximal expansion, we obtain the total volume of the Universe calculated in problem at this same moment. At later times $\eta>\pi$ we can see all of the Universe, moreover some points from different directions, so the formula is not applicable. c) The volume that we are observing directly differs from the calculated above as we see the distances between objects as they were at the moment of emission, not as they are "now", so one $a(t)$ shoulf be left inside the integral. Otherwise following the same steps, we arrive to \[V_{c}=4\pi (a_{0}\alpha_{\star})^{3} \int\limits_{0}^{\eta_{0}}d\eta\, (1-\cos\eta)^{3}\sin^{2}\eta.\] In more rigorous terms: consider the $4$-volume $\Omega$ filled with worldlines of all particles which we are currently observing (i.e. the ones the light from which took less time to arrive to us than the age of the Universe). The previous volume $V_{b}$ was the spatial volume that the observed matter occupies "at present", i.e. it is the volume of the section $t=t_{0}$ of $\Omega$. The volume $V_{c}$ is the 3-volume of the section of $\Omega$ by the light "cone" directed to the past with the vertex at the observer (it is actually a cone only in Minkowski spacetime, and is quite deformed for Friedmannian spaces).
Different problems
Problem 29: open dusty Universe
Find the solution of Friedman equations for spatially open ($k=-1$) Universe filled with dust in the parametric form $a(\eta)$, $t(\eta)$.
The solution differs from the one for $k=+1$ (problem) only by the sign of $k$. Likewise the first Friedman equation is rewritten as \[\frac 1{a^4}\left(\frac{da}{d\eta} \right)^2 =\frac{2a_{\star}}{a^3}+\frac{1}{a^2}, \quad\text{where}\quad a_{\star}=\frac{8\pi G \rho_{0}}{3},\] and on integration we obtain \[ \eta=\int\limits_{0}^{a/a_{\star}} \frac{dx}{\sqrt{2x+x^{2}}} =\text{arcosh}\Big(1+\frac{a}{a^{\star}}\Big),\] so trigonometric functions are replaced by the hyperbolic ones and \begin{align}\label{dyn-open} a(\eta)&=a^{\star}(\cosh\eta-1),\\ t(\eta)=\int a d\eta&=a^{\star}(\sinh\eta-\eta), \qquad \eta\in(0,\infty).\nonumber \end{align}
Problem 30: density evolution
Suppose the density of some component in a spatially flat Universe depends on scale factor as $\rho(t) \sim a^{-n}(t)$. How much time is needed for the density of this component to change from $\rho_1$ to $\rho_2$?
Let $\rho(t) = b a(t)^{-n}$, then expressing $a$ and $\dot{a}$ in the first Friedman equation through $\rho$ and $\dot{\rho}$, we are led in result to equation \[\frac{d\rho}{dt}=n\sqrt{ \frac{8\pi G}{3}}\rho^{3/2},\] and after integration to \[ \Delta t =\frac{1}{n} \sqrt{\frac{3}{8\pi G}} \int\limits_{\rho_1}^{\rho_2}\!d\rho\;\rho^{-3/2} =\frac{2}{n}\sqrt{ \frac{3}{8\pi G}}\;\; \big(\sqrt{\rho_1}-\sqrt{\rho_2}\big).\]
Problem 31: $q[H(t)]$
Using the expression for $H(t)$, calculate the deceleration parameter for the cases of domination of
a) radiation,
b) matter.
As \[\frac{d}{dt}\frac{1}{H}=\frac{d}{dt}\frac{a}{\dot{a}} =1-\frac{a\ddot{a}}{a^2}=1+q,\] from \[H=\frac{2}{3(1+w)t}\] we obtain the formula already derived above (\ref{dyn-q(w)}) \[q=\frac{3(1+w)}{2}-1=\frac{1+3w}{2}.\] Then a) for radiation $q=\frac{1}{2}$; b) for matter $q=1$.
Problem 32: effective EoS for multi-component Universe
Consider a Universe consisting of $n$ components, with equations of state $p_{i}=w_{i}\rho_{i}$, and find $w_{tot}$, the parameter of the equation of state $p_{tot}=w_{tot}\rho_{tot}$.
By definition \[p_{tot}=w_{tot}\rho_{tot},\] so \begin{equation}\label{w_tot_i} w_{tot}=\frac{\sum\limits_i w_i \rho_i}{\sum\limits_i \rho_i}=\frac{\sum\limits_i w_i \Omega_i}{\sum\limits_i \Omega_i}. \end{equation} For a flat Universe $\sum\Omega_{i}=\Omega=1$, and in case of two-component medium \begin{equation}\label{w_tot_d_m} w_{tot}=\Omega_{m}w_{m}+\Omega_{d}w_{d}. \end{equation}
Problem 33: relative densities
Derive the equations of motion for relative densities $\Omega_{i}=\rho_{i}/\rho_{cr}$ of the two components comprising a spatially flat two-component Universe, if their equations of state are $p=w_i\rho$, $i=1,2$.
First let us note that from the conservation laws for each of the components separately and both of them together \begin{align*} \dot{\rho}_{i}&=-3H(1+w_{i})\rho_{i};\\ \dot{\rho}_{tot}&=-3H(1+w_{tot})\rho_{tot}. \end{align*} On substitution of $\dot{\rho}_{i}$ into the time derivative of $\Omega_{i}=\rho_{i}/\rho_{cr}$ we get \[\frac{1}{H}\dot{\Omega}_{i}=\frac{1}{H} \frac{\dot{\rho}_{i}\rho_{tot}- \rho_{i}\dot{\rho}_{tot}}{\rho_{tot}^{2}} =3\Omega_{i}(w_{tot}-w_{i}).\] For a two-component flat Universe we can use the result (\ref{w_tot_d_m}) for $w_{tot}$, thus \[\dot{\Omega}_{m}=-\dot{\Omega}_{r} =3H\Omega_{m}\Omega_{r}(w_{r}-w_{m}).\]
Problem 34: EoS for non-relativistic gas
Suppose a Universe is initially filled with a gas of non-relativistic particles of mass density $\rho_{0}$, pressure $p_{0}$, and $c_p/c_v=\gamma$. Construct the equation of state for such a system.
Let us rewrite the conservation law in the Friedmannian Universe \[\dot{\rho}+3H(\rho+p)=0\] (here $c=1$) in the form \[\dot{\rho}+\frac{\dot{V}}{V}(\rho+p)=0,\] and passing to differentiation by pressure $p$ instead of time $t$, as \[\frac{d\rho}{dp}+\frac{1}{V}\frac{dV}{dp} (\rho+p)=0.\] For adiabatic expansion \[pV^\gamma = const\quad \Rightarrow\quad V\sim p^{-1/\gamma}\quad \Rightarrow\quad \frac{1}{V}\frac{dV}{dp}=-\frac{1}{\gamma p}.\] Plugging this into the conservation law, we get a differential equation for $\rho(p)$: \[\gamma p\cdot \rho'=\rho+p.\] Its solution is %\[\rho=\rho _m + \frac{p}{\gamma-1}.\] \[\rho(p)=\frac{p}{\gamma-1}+C\,p^{1/\gamma}.\] Using the initial conditions then \[\rho(p)=\frac{p}{\gamma-1} +\Big(\rho_{0}-\frac{p_{0}}{\gamma-1}\Big) \left(\frac{p}{p_{0}}\right)^{1/\gamma}.\]
Problem 35: Newtonian derivation of critical density
Derive the expression for the critical density $\rho_{cr}$ from the condition that Hubble's expansion velocity equals the second cosmic velocity (escape velocity) $v=\sqrt{2gR}$.
Let us consider the spherical region of radius $R$ with density $\rho_{cr}$. Putting down the escape velocity as $\dot R$, we obtain \[\dot R^2 = 2\frac{G\rho _{cr}\cdot\frac{4}{3}\pi R^3}{R^2}R.\] Then taking into account the definition of $H = \dot R/R$, \[\rho_{cr}=\frac{3H^2}{8\pi G}.\]
X-component
Problem 36: dust plus something else
Suppose the Universe is filled with non-relativistic matter and some substance with equation of state $p_X=w\rho_X$. Find the evolution equation for the quantity $r \equiv \frac{\rho_m}{\rho _X}$.
From $r=\rho_{m}/\rho_{X}$ \[\dot r = \frac{{\dot \rho}_m}{\rho _X} - {\dot \rho }_X{\rho_m}{\rho _X^2}.\] Then on substitution of ${\rho _m}$ and ${\rho _X}$ from the corresponding conservation laws, we get \[\dot r = 3Hwr.\]
Problem 37: deceleration parameter
Express the deceleration parameter through the ratio $r$ for the conditions of the previous problem.
Similarly to problem, on substitution of the right hand parts of the Friedman equations into the numerator and denominator, we get \[q = - \frac{\ddot{a}/a}{H^2} = \frac{\frac{4\pi G}{3}(\rho + 3p)}{\frac{8\pi G}{3}\rho } = \frac{1}{2}\frac{\rho_m + \rho_X + 3w\rho _X}{\rho_m +\rho _X} =\frac{1}{2} + \frac{3w}{2(1+r)}.\] Another way: \[q = - 1 + \frac{d}{dt}\left( \frac{1}{H} \right) = - 1 - \frac{\dot H}{H^2};\] \[\dot H = - 4\pi G(\rho + p), \quad {H^2} = \frac{8\pi G}{3}\rho.\]
Problem 38: $\rho_{X}\propto H^2$
Let a spatially flat Universe be filled with non-relativistic dust and a substance with equation of state $p_{X}= w\rho_{X}$. Show that in case $\rho_{X}\propto H^2$, the ratio $r =\rho_{m}/\rho_{X}$ does not depend on time.
The Friedman equations can be written as \[3{H^2} = 8\pi \rho - 3\frac{k}{a^2},\] \[\dot H = - 4\pi G\left( {\rho + p} \right) + \frac{k}{a^2}.\] Then \[2\frac{{\dot{H}}}{H} =-3H\left( 1+\frac{p}{\rho } \right) -\frac{k}{{{a}^{2}}H}\left( 1+3\frac{p}{\rho } \right).\] Combining this with the conservation equation for $\rho_X$, we get \[\frac{\dot{\rho}_X}{\rho_X} -2\frac{\dot{H}}{H} =-\frac{3Hwr}{1+r}+\frac{k}{{{a}^{2}}H} \left( 1+3\frac{w}{1+r} \right)\] In the considered case (see problem) $\dot{r}=3Hwr$, so we arrive to \[\dot{r}=-\left( 1+r \right)\left[ \frac{{{{\dot{\rho }}}_{X}}}{{{\rho }_{X}}}-2\frac{{\dot{H}}}{H}-\frac{k}{{{a}^{2}}H}\left( 1+3\frac{w}{1+r} \right) \right].\] If ${{\rho }_{X}}\propto {{H}^{2}}$, then \[\dot{r}=(1+r) \frac{k}{{{a}^{2}}H}\left( 1+3\frac{w}{1+r} \right).\] Thus we obtain immediately that for a flat Universe $\dot{r}=0$.
Problem 39: $r(q)$
Show that for the model of the Universe described in the previous problem the parameter $r$ is related with the deceleration parameter as \[\dot{r}=-2H\frac{\Omega_{curv}}{\Omega_X}q.\]
Combining \[\dot{r}=(1+r)\frac{k}{{{a}^{2}}H}\left( 1+3\frac{w}{1+r} \right)\] with \[q=\frac{1}{2}\left( 1+\frac{k}{{{a}^{2}}{{H}^{2}}} \right)\left( 1+3\frac{w}{1+r} \right)\] we find \[\dot{r}=2\left( 1+r \right)\frac{k}{{{a}^{2}}H}\frac{q}{1+\frac{k}{{{a}^{2}}{{H}^{2}}}}\] Using the relative densities \[{{\Omega }_{m}}=\frac{8\pi G}{3{{H}^{2}}}{{\rho }_{m}},\quad {{\Omega }_{X}}=\frac{8\pi G}{3{{H}^{2}}}{{\rho }_{X}},\quad {{\Omega }_{curv}}=-\frac{k}{{{a}^{2}}{{H}^{2}}}\] we can transform the combination $1+r$ to \[1+r=1+\frac{{{\rho }_{m}}}{{{\rho }_{X}}}=\frac{1-{{\Omega }_{curv}}}{{{\Omega }_{X}}}=\left( 1+\frac{k}{{{a}^{2}}{{H}^{2}}} \right)\frac{1}{{{\Omega }_{X}}}\] Finally \[\dot{r}=-2H\frac{{{\Omega }_{curv}}}{{{\Omega }_{X}}}q\] Yet again we confirm that for the considered model in the flat case $\dot{r}=0$.
Problem 40: $r$ in open and closed cases
Show that in the model of the Universe of problem, in case $k=+1$ and $q>0$ (decelerated expansion) $r$ increases with time, in case $k=+1$ and $q<0$ (accelerated expansion) $r$ decreases with time, and for $k=-1$ vice-versa.
The result immediately follows from the ralation obtained in the previous problem \[\dot{r}=-2H\frac{{{\Omega }_{curv}}} {{{\Omega }_{X}}}q\] and definition \[{\Omega }_{curv}=-\frac{k}{{{a}^{2}}{{H}^{2}}}.\]
Power-law cosmology
The following problems on power-law cosmology are inspired by Kumar [1].
Problem 41: power-law cosmologies
Let us consider a general class of power-law cosmologies described by the scale factor \[a(t) =a_{0}\Big(\frac{t}{t_0}\Big)^\alpha,\] where $t_0$ is the present age of theUniverse and $\alpha$ is a dimensionless positive parameter. Show that:
1) the scale factor in terms of the deceleration parameter may be written as \[a(t) =a_{0}\Big(\frac{t}{t_0}\Big)^{1/1 + q}, \quad\text{i.e.}\quad \alpha=\frac{1}{1+q}.\]
2) the expansion of the Universe is described by Hubble parameter \[H=\frac{1}{(1+q)\;t}\] or in terms of redshift \[H(z)=H_{0}(1+z)^{1+q}.\]
As $H=\dot{a}/a=\alpha/t$, using the definition for the deceleration parameter, we get \[q=-\frac{\ddot{a}/a}{H^2}=\frac{1-\alpha}{\alpha},\] so \[\alpha=\frac{1}{1+q},\qquad H=\frac{1}{(1+q)\;t}.\]
Problem 42: age of the Universe
In the power-law cosmology find the age of the Universe at redshift $z$.
In the general case, using the relation $(1+z)=1/a$, we can relate the age of the Universe to the expansion history as \[t=\int dt=\int \frac{da/a}{H} =\int\limits_{z}^{\infty} \frac{dz}{(1+z)H}.\] Then using the result of the previous problem), on integration we find \[t(z)=\frac{\alpha/H_{0}}{(1+z)^{1/\alpha}}.\]
Problem 43: luminosity distance
For the power-law cosmology find the luminosity distance between the observer and the object with redshift $z$.
The luminosity distance is \[{D_L}(z) = (1 + z)\int_0^z \frac{dz'}{H(z')} = \frac{1 + z}{H_0}\frac{\alpha }{1-\alpha} (1 + z)^{\frac{\alpha-1}{\alpha}}\Big|_{0}^{z} =\frac{H_{0}^{-1}}{q} (1+z)\big[1-(1+z)^{1-q}\big].\]
Problem 44: a "softer" version of the cosmological evolution
The power law cosmological evolution $a(t)\propto t^{\beta }$ leads to the Hubble parameter $H(t)\propto 1/t$. Consider a "softer" version of the cosmological evolution given by the law
\[H(t)=\frac{S}{t^{\alpha } } \]
where $S$ is a positive constant and $0<\alpha <1$. Analyze the dynamics of such model at $t\to 0$.
(F.Cannata, A.Kamenshchik, D.Regoli, arXiv:0801.2348).
Integrating $\frac{d}{dt} \left(\ln a\right)=\frac{S}{t^{\alpha } } $ we obtain \[\ln \left(\frac{a(t)}{a(0)} \right)=\frac{S}{1-\alpha } t^{1-\alpha } \] At $t=0$ singularity is present, but it is different from the traditional Big Bang singularity. If $t\ne 0\, (t>0)$, the right hand side is finite and hence one cannot have $a\left(0\right)=0$ in the left hand side. Then $a(0)>0$, while \[\dot{a}(t)=a(0)\frac{S}{t^{\alpha } } \exp \left(\frac{S}{1-\alpha } t^{1-\alpha } \right)\mathop{\to }\limits_{t\to 0} \, \infty. \] This type of singularity received the name of "soft Big Bang singularity" because the cosmological scale factor is finite (and non-zero) while its time derivative, the Hubble variable and the scalar curvature are singular. It is interesting to note that when $t\to \infty $ both $a(t)$ and $\dot{a}(t)$ tend to infinity, but they do not encounter any cosmological singularity because the Hubble variable and its derivatives tend to zero.